Protocols for Integrity Constraint Checking in Federated Databas

1 . Distributed and Parallel Databases, 5, 327–355 (1997) c 1997 Kluwer Academic Publishers. Manufactured in The Netherlands. Protocols for Integrity Constraint Checking in Federated Databases* PAUL GREFEN grefen@cs.utwente.nl Dept. of Computer Science, University of Twente, 7500 AE Enschede, The Netherlands JENNIFER WIDOM widom@cs.stanford.edu Dept. of Computer Science, Stanford University, Stanford, CA 94305, USA Received October 8, 1996; Accepted April 25, 1997 Recommended by: Elisa Bertino Abstract. A federated database is comprised of multiple interconnected database systems that primarily operate independently but cooperate to a certain extent. Global integrity constraints can be very useful in federated databases, but the lack of global queries, global transaction mechanisms, and global concurrency control renders traditional constraint management techniques inapplicable. This paper presents a threefold contribution to integrity constraint checking in federated databases: (1) The problem of constraint checking in a federated database environment is clearly formulated. (2) A family of protocols for constraint checking is presented. (3) The differences across protocols in the family are analyzed with respect to system requirements, properties guaranteed by the protocols, and processing and communication costs. Thus, our work yields a suite of options from which a protocol can be chosen to suit the system capabilities and integrity requirements of a particular federated database environment. Keywords: integrity control, global integrity constraint, federated database, protocol 1. Introduction The integration of multiple database systems has become one of the most important topics in both the research and commercial database communities. Information servers are being developed that provide integrated access to multiple data sources. Legacy database systems and applications are being coupled to form enterprise-wide information systems. Large workflow management applications require the routing of information through multiple, autonomous local systems. The integration of autonomous database systems into loosely- coupled federations requires the development of novel database management techniques specific to these environments. Many concepts and techniques from centralized or tightly- coupled distributed databases are not directly applicable in a federated environment. One important issue in federated database systems is checking integrity constraints over data from multiple sites in the federation. Integrity constraints specify those states of the (global) database that are considered to be semantically valid. In a federated environment, integrity constraints might specify that replicated information is not contradictory, that * This work was supported at Stanford by ARPA Contract F33615-93-1-1339 and by equipment grants from Digital Equipment and IBM Corporations. A short preliminary version of this work appeared in the proceedings of the 1996 CoopIS conference. 

2 .328 GREFEN AND WIDOM information is not duplicated, that certain referential integrity constraints hold, or that some other condition is true over the data in multiple databases. Below, we describe an example scenario involving cooperative hospital information systems with cross-system constraints. In traditional centralized or tightly-coupled distributed databases, transactions form the cornerstone of integrity constraint checking: Before a transaction commits, it ensures that all integrity constraints are valid. If a constraint is violated, then the transaction may be aborted, the constraint may be corrected automatically, or an error condition may be raised [14]. Unfortunately, the lack of inter-site transaction mechanisms in federated databases renders traditional constraint checking mechanisms inapplicable. This paper addresses the problem of integrity constraint checking in federated databases; we make a threefold contribution. First, the constraint checking problem is formulated in the specific context of federated databases. In particular, an alternative notion of con- straint checking correctness must be defined, since the transaction-based approach from traditional environments is inappropriate. Then a family of constraint checking protocols is developed along a number of protocol “dimensions.” Finally, the protocols in the family are analyzed and compared with respect to the requirements of the component database systems, the constraint checking properties guaranteed by the protocols, and the processing and communication costs. By providing a family of protocols, rather than a single proto- col, we permit a protocol in the family to be chosen and tailored for the capabilities and requirements of a particular federated database environment. 1.1. Related Work Most work addressing the problem of integrity constraint checking in multidatabase en- vironments has considered tightly-coupled distributed databases in which global queries, global transactions, and global concurrency control are present, e.g., [13, 24, 28]. Since these approaches rely on global services that typically are unavailable in federated databases, they are inappropriate for the environment we consider. Note that some approaches focus on relaxing the traditional notion of transaction serializability for constraints in distributed environments, e.g., [3, 11, 12], but some level of locking and global query facilities is still expected. A few recent papers have addressed the issue of monitoring constraints in loosely-coupled, distributed, and sometimes heterogeneous database environments. One class of work in- volves local constraint checking—deriving tests whose success over one database implies the validity of a multidatabase constraint [2, 17, 18]. Local tests optimize the constraint checking process, but they still require a conventional (non-local) method when the local test fails. As will be seen, in this paper we develop protocols that integrate local check- ing with non-local methods. In [7], a framework and toolkit are described for constraint management in loosely-coupled, highly heterogeneous environments. The focus in [7] is on maintaining constraints across systems that have varying capabilities and varying “will- ingness” to participate in constraint checking protocols, on describing the timing properties associated with constraint checking, and on notions of “conditional consistency” that are weaker than the form of consistency we consider. 

3 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 329 The issue of maintaining consistency of replicated data across loosely-coupled, seman- tically heterogeneous databases has been considered in, e.g., [9, 10, 27]. Our constraint checking problem can be seen as a special case (or first step) of that consistency maintenance problem. In [9], a method is described that relies on active rules and persistent queues. The approach is similar to the simplest case in our family of protocols. Similar issues are addressed in [27], but no specific protocols are provided. In [10], an implementation mechanism based on active rules is proposed for maintaining replication consistency. A related problem is that of maintaining views over distributed data in loosely-coupled systems, addressed in [31] in the context of data warehousing. In [31], algorithms are presented for handling the anomalies that arise when materialized views are refreshed in an asynchronous manner. The algorithms rely on view definitions and compensating queries, and thus are not directly applicable to our problem. However, they may become relevant as we extend our protocols to handle more complex constraints or to incorporate consistency repair. Finally, we note that in the field of distributed (operating) systems there has been consid- erable work in the area of snapshots and consistent global states; see e.g., [8, 5]. Although this work appears highly related to the problem we are addressing, there are two significant differences: • The conditions to be evaluated in the distributed system setting are stable, meaning that once a condition becomes valid, it stays valid. This property is not true of database integrity constraints; in fact, our goal is to track constraints as they move from validity to invalidity and vice-versa. • Protocols for the distributed system setting are designed to obtain some (any) global state, but not to obtain all global states. In contrast, to effectively monitor database constraints it is necessary to monitor all global states, or at least a subset of those states corresponding to consistency “checkpoints” (see Section 2.1). 1.2. Structure of the Paper The structure of this paper is as follows. Section 2 formalizes the problem of integrity constraint checking in federated databases. It provides necessary concepts and definitions, describes the basic system architecture we address, discusses implementation aspects, and defines the class of integrity constraints we deal with. In addition, a simple example application is introduced. Section 3 presents the family of constraint checking protocols we have developed. Section 4 analyzes the family of protocols presented in Section 3. First, the “design space” for protocols is further inspected. Then the various protocols are compared with respect to their requirements, properties, and costs. Finally, we describe how the protocols can be extended to more general architectures. In Section 5 we conclude and discuss future work. 

4 .330 GREFEN AND WIDOM 2. Preliminaries This section presents preliminary material for the remainder of the paper. First, the concepts used in our work are defined formally. Next, we present the basic architecture we consider— a federation of two autonomous, relational databases, each with a component for constraint checking—and we discuss implementation aspects of the architecture. Then we describe the class of integrity constraints we consider. The section ends with the description of an example application that is used to motivate our work. Note that although we cast our work in the context of federations of relational databases, the relational restriction is introduced primarily for concreteness and clarity. All of our def- initions, protocols, and analyses adapt easily to other data models, as well as to federations involving multiple data models. 2.1. Concepts and Definitions We start with our definition of a federated relational database system. Definition 1. A federated relational database system F is a set of n interconnected au- tonomous database systems {S1 , . . . , Sn }. Each autonomous database system Si ∈ F hosts a local database Di with schema Di . A local database Di consists of relations R1i , . . . , Rni i with schemata Ri1 , . . . , Rini . The set of all relation schemata Rij in F is called the global database schema G of F . ✷ A federated database system is often constructed by coupling a number of preexisting local database systems, i.e., it is designed in a bottom-up fashion from a number of independent local database designs. In the following, we assume a global clock so that we can refer to global times in defining certain concepts. The global clock is used for concept definition only—it is not a require- ment of the federated database systems we consider. Also for definitional purposes, we assume that each local database processes its updates in the context of local transactions. However, many of the constraint checking protocols we present are also applicable to local systems that do not support transactions. Definition 2. The user-observable state of a relation Rji at global time t is the state of Rji reflecting all and only those local update transactions committed before t at site Si . ✷ Definition 3. The global state G of a global database schema G at global time t is the set of user-observable states of all relations in G at global time t. ✷ Due to the lack of global transactions in a federated environment, it can be difficult or impossible to observe the state of a global database at a single global time. If an application or protocol does not read local states simultaneously, it may observe a global state that has never actually existed. We call such an observed state a phantom state. Definition 4. A phantom state Φ of a global database schema G observed by application A at time t2 as a consequence of a request (or set of requests) by A at time t1 is a set of states 

5 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 331 of all relations in G such that there exists no t3 where t1 < t3 < t2 and the global state of G at time t3 is Φ. ✷ The relevance of phantom states will become clear when we develop our protocols in Sec- tion 3. Next, we turn to the definition of global integrity constraints, and we formalize our notion of correctness in constraint checking. Definition 5. A global integrity constraint I is a boolean expression over a global database schema G, i.e., a function I: G → {true, f alse}. A global integrity constraint cannot be expressed over a local database schema Di ∈ G (otherwise the constraint would be local, not global). A constraint checking protocol for I is an algorithm for evaluating I. ✷ In centralized database systems or tightly-coupled distributed database systems, the tran- sitions between database states are determined by transactions—atomic operations whose intermediate states have no semantics beyond the transaction. Consequently, in these en- vironments, integrity constraints generally are required to hold in the states immediately preceding and following each transaction. Since federated environments consist of multiple, autonomous database systems lacking global transactions, we must rely on other concepts to determine the global database states that should satisfy the integrity constraints. We define a notion of global states in which the federated system is “at rest.” These quiescent states correspond roughly to the before and after transaction states in traditional database systems, and are the states in which we want to ensure that integrity constraints are not violated. Definition 6. A federated database system F is in a quiescent state at time t if all local up- date transactions submitted before t have committed, and all constraint checking protocols triggered by any updates before t have completed. ✷ It is important to note that, similar to the before and after transaction states in traditional systems, quiescent states may not physically exist. However, the logical notion of such states is appropriate for defining the correctness of integrity constraint checking. Two im- portant properties of our constraint checking protocols are defined with respect to quiescent states: safety and accuracy. Definition 7. Consider a global database schema G and a global integrity constraint I over G. A constraint checking protocol for I is safe if the transition from any quiescent global database state G0 ∈ G that satisfies I to any other quiescent state G1 ∈ G that does not satisfy I always results in the protocol raising an alarm.1 ✷ Definition 8. Consider a global database schema G and a global integrity constraint I over G. A constraint checking protocol for I is accurate if, after any quiescent global database state G0 at time t0 , a protocol-generated alarm at time t2 implies the existence of a global database state G1 at time t1 such that t0 < t1 ≤ t2 and G1 does not satisfy I. ✷ That is, a protocol is safe if it detects every transition to a quiescent state in which the constraint becomes violated. We assume that safety is required of any constraint checking protocol that will be useful in practice. A safe protocol may be “pessimistic,” however, in the sense that it raises too many alarms. (For example, a protocol that constantly raises 

6 .332 GREFEN AND WIDOM alarms is, in fact, safe.) A protocol is accurate if, whenever an alarm is raised, there is in- deed a state in which the constraint is violated. Although accuracy is a desirable feature of a constraint checking protocol, it is not always necessary—in some environments a limited number of “false alarms” is tolerable. Our last definition involves the representation of database updates. Definition 9. Consider a relation R in a local database system of a federation. When R is modified, we use ∆R to denote all modified tuples (inserted, deleted, or updated), ∆+ R to denote all new tuple values (inserts and after-images of updates), ∆− R to denote all old tuple values (deletes and before-images of updates), and ∆0 R to denote all unmodified tuples. We refer to all forms of ∆’s as delta sets. We assume that delta sets correspond to the modifications performed by a single local transaction, however other update granularities can be used without affecting our protocols. ✷ 2.2. Basic Architecture and Assumptions Figure 1 depicts the basic architecture we address for integrity constraint checking in feder- ated databases. To develop our protocols we consider the restricted case of two databases, each containing a single relation. In practice, most multi-site constraints involve only two databases or can be reformulated as an equivalent set of two-site constraints. However, our protocols can be generalized to handle constraints over more than two sites—see Section 4.3 for a discussion. Generalizing to more than one relation per site is straightforward. Figure 1. Basic architecture In the diagram, DBR and DBS denote two local database systems managing relations R and S, respectively. Connected to the local database systems are constraint managers, CMR and CMS in the diagram. There is one constraint manager for each local database— each constraint manager handles the global constraints that may be invalidated by operations on its corresponding database. (Consequently, each global constraint is replicated at every site containing data involved in the constraint.) We assume that any local constraints are 

7 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 333 managed within the appropriate database system using traditional mechanisms (see, e.g., [14]). The constraint manager is notified of all changes (∆’s) to the local database that may violate a constraint. For convenience, we may assume that delta notifications are sent at the end of each local update transaction; however, as mentioned earlier, any granularity of delta notification can be handled by our protocols. Of particular importance is that we do not generally assume that delta notifications or subsequent constraint checking protocols occur as part of a local transaction, and constraint managers need not be tightly coupled with their corresponding database systems. The database systems in the federation are autonomous, in that global queries, global transactions, and global concurrency control mechanisms are not available. The fact that global query mechanisms are not assumed means that we deal with loosely-coupled fed- erated databases [1]. The approach presented in this paper is, however, also applicable in federated database systems where global query facilities are available, i.e., tightly-coupled federated databases. A message-passing interface connects the two sites. This interface allows the constraint managers to exchange messages in a cooperative fashion, and may allow a constraint man- ager to send requests directly to the remote database system. 2.3. Implementation Aspects An essential aspect of implementing the above architecture is supporting the delta no- tification mechanism by which database systems in a federation communicate with their respective constraint managers. Various implementation schemes can be used to realize this mechanism, including active database rules, passive database rules, database triggers, and polling. Active database rules follow the event-condition-action (ECA) paradigm [30]. These rules can be used to implement the delta notification mechanism in a straightforward way: WHEN relevant update has occured IF global constraint possibly violated THEN notify constraint manager Clearly, the event and condition (WHEN and IF clauses) are “pessimistic,” since the actual violation of a global constraint cannot in general be determined by the local database system. For database systems that do not include an active rule manager, passive rules can be used: Passive rules are processed by a transaction modification component built on top of a conventional database system [16, 21]. While active rules respond to the actual effects of updates to the database, passive rules act on the mere execution of an update operation, regardless of its effects. Thus, the triggering behaviour of passive rules is more pessimistic, but their implementation and execution costs can be lower. Database triggers, as supported by many recent commercial database systems [30], can be used in a similar way to the rules described above. Usually, their abstraction level is lower than that of active or passive rules, making the implementation of the notification mechanism slightly more complex. 

8 .334 GREFEN AND WIDOM Finally, in cases where no triggering mechanism is available at the database system level (e.g., in strictly closed legacy systems), delta notification must be handled by the constraint manager using a polling mechanism. In this approach, the constraint manager polls the local database system at a certain frequency to detect when changes have occurred. The database system has to maintain delta sets in this case that contain the relevant changes to the database. The constraint manager must be able to read these delta sets and empty them after processing. Certainly this approach is the least desirable from both a performance and architecture standpoint, but it is necessary in the case of closed systems with no notification mechanism. 2.4. Integrity Constraints In this paper we consider constraints over two relations, R and S. In particular, we consider constraints that can be evaluated incrementally. By incremental, we mean that if the con- straint is valid initially, and if one of the relations is changed—relation R, say—then the constraint can be reevaluated by considering only the changes to R (∆R) and the relation S. This class of constraints includes many of the most common constraint types, such as refer- ential integrity and mutual exclusion. See the earlier technical report version of this paper [19] for a complete definition of the class of constraints considered. The problem of incre- mental constraint checking has been studied extensively (see, e.g., [4, 13, 22, 26, 25, 29]). The protocols we present can be extended in a straightforward way to constraints over more than two relations; the two-relation restriction is adopted for clarity and brevity only. We assume that constraints are expressed as queries, where the constraint is satisfied iff the query result is empty. This is a common and convenient formulation [14, 17, 19], equivalent to expressing constraints as logical formulae. Hence, we denote a constraint C over relations R and S as a query Q(R, S). A query to check C incrementally with respect to changes on R is denoted Q (∆R, S), where again the constraint is satisfied iff the query result is empty (assuming the constraint held before R was modified [4, 25, 29]). Q (R, ∆S) is a similar query to handle modifications to S. 2.5. Example Application Suppose we have two hospitals, HA and HB , in two neighboring cities. Each hospital has its own database system, SA and SB respectively. When the hospitals start a cooperation in which they share physicians, it is decided to connect the two existing database systems into a federation to be able to also share information. The relations of the resulting federated database are shown in Table 1. Both hospitals keep a local record of their patients, and each patient has a reference to his or her physician. To avoid duplication of information, the local physician administrations have been merged into a central administration at hospital HA . The hospitals enforce a rule that each patient can be registered at only one hospital. In addition, all patients must have a registered physician. These rules lead to the following 

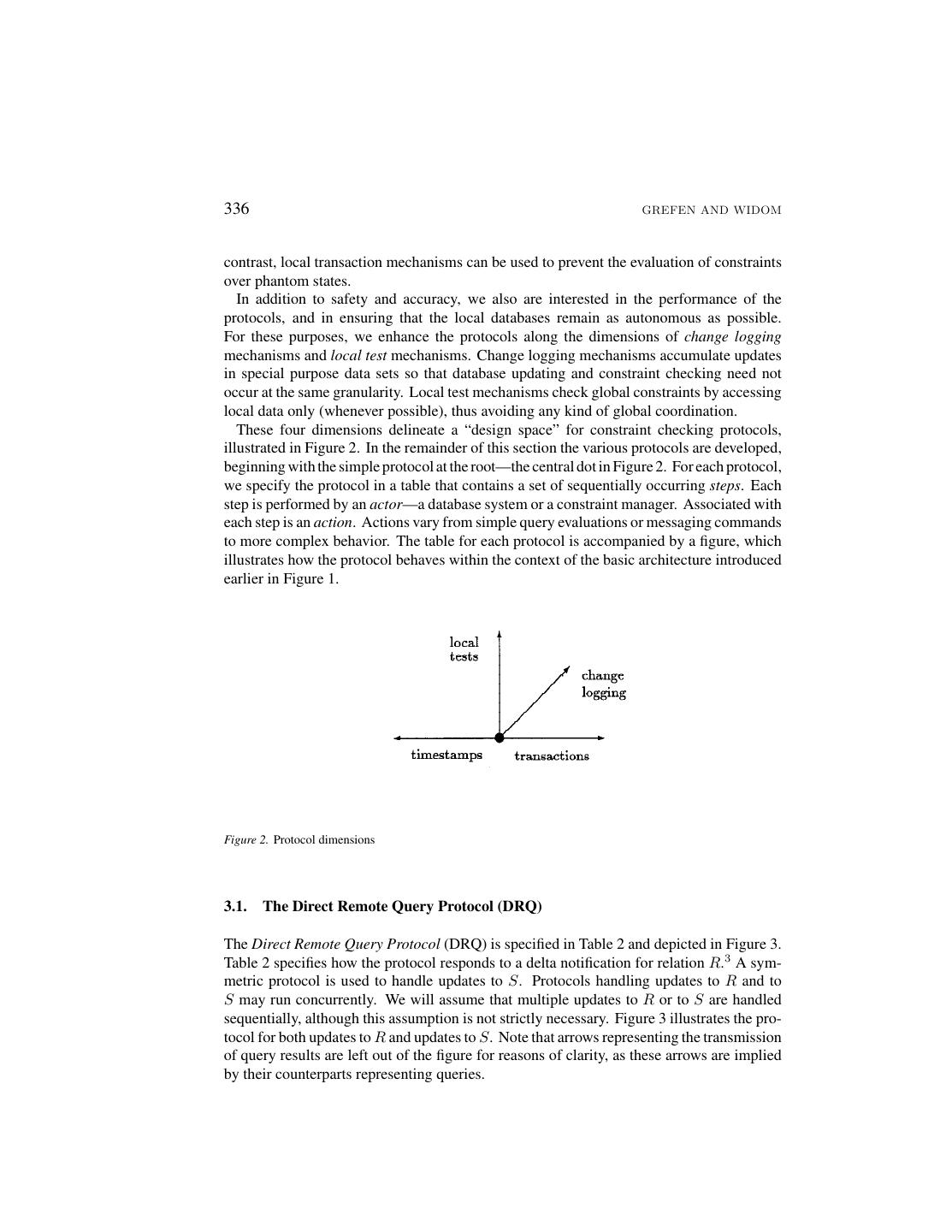
9 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 335 Table 1. Example database relations system relation SA P atientsA (SSN, N ame, Address, DateOf Birth, P hysician) SB P atientsB (SSN, N ame, Address, P hone, Sex, P hysician) SA P hysicians(P hN, N ame, P hone) three integrity constraints, expressed as relational algebra queries. (Recall that the constraint is satisfied when the result of the query is empty.) C1 : P atientsA ✶SSN =SSN P atientsB C2 : ΠP hysician P atientsA − ΠP hN P hysicians C3 : ΠP hysician P atientsB − ΠP hN P hysicians Constraints C1 and C3 are global constraints, since they involve relations at two different sites. Constraint C2 is a local constraint, so it can be enforced at its local site (SA ) using standard methods. Constraints C1 and C3 can each be “factored” into two incremental global constraints [29], dealing with relevant updates to each of the two relations: C1A : ∆+ P atientsA ✶SSN =SSN P atientsB C1B : P atientsA ✶SSN =SSN ∆+ P atientsB C3B : ΠP hysician ∆+ P atientsB − ΠP hN P hysicians C3A : ΠP hysician P atientsB ∩ ΠP hN ∆− P hysicians 3. The Family of Protocols As motivated earlier, conventional integrity constraint checking methods are not applicable in federated environments, so alternative protocols must be designed. In the remainder of this paper we develop and analyze a family of constraint checking protocols suitable for federated databases. The members of this family have different requirements, different properties, and different performance characteristics. The “root” of the family is a very simple protocol, described in Section 3.1. Although this protocol is safe, it is inaccurate because it may evaluate constraints over phantom states (recall Definition 4). In Sections 3.2–3.8 we enhance the simple protocol along a number of different dimensions to obtain more useful protocols. In designing the protocols, we are especially interested in the safety and accuracy prop- erties as defined in Section 2.1. The root of the protocol family is safe, and so are all of the protocols derived from it. To obtain accuracy, we enhance the root protocol along the dimensions of timestamping mechanisms and local transaction mechanisms. Timestamp- ing mechanisms annotate delta sets and query results with global timestamps,2 enabling the algorithm to detect when phantom states may have been used in constraint evaluation. In 

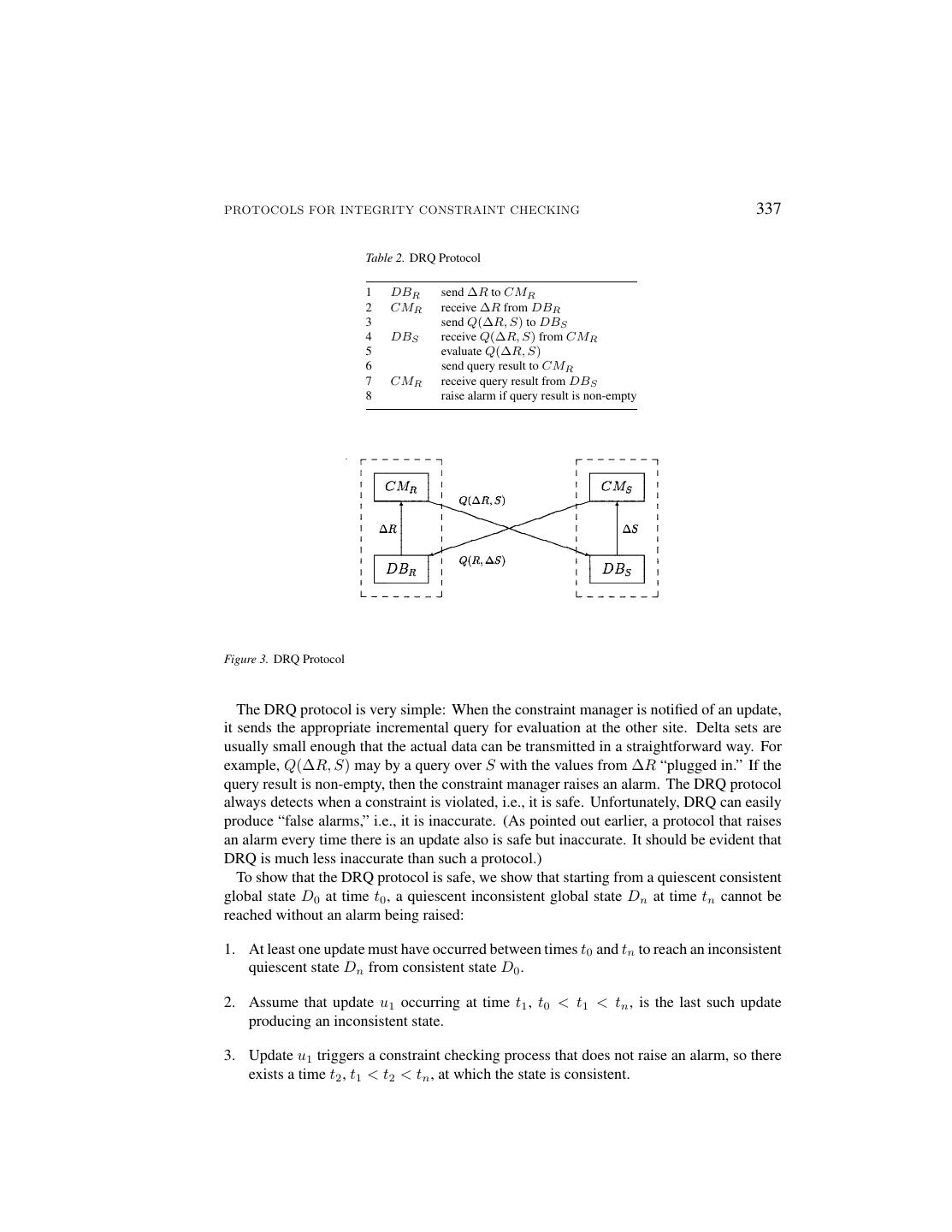
10 .336 GREFEN AND WIDOM contrast, local transaction mechanisms can be used to prevent the evaluation of constraints over phantom states. In addition to safety and accuracy, we also are interested in the performance of the protocols, and in ensuring that the local databases remain as autonomous as possible. For these purposes, we enhance the protocols along the dimensions of change logging mechanisms and local test mechanisms. Change logging mechanisms accumulate updates in special purpose data sets so that database updating and constraint checking need not occur at the same granularity. Local test mechanisms check global constraints by accessing local data only (whenever possible), thus avoiding any kind of global coordination. These four dimensions delineate a “design space” for constraint checking protocols, illustrated in Figure 2. In the remainder of this section the various protocols are developed, beginning with the simple protocol at the root—the central dot in Figure 2. For each protocol, we specify the protocol in a table that contains a set of sequentially occurring steps. Each step is performed by an actor—a database system or a constraint manager. Associated with each step is an action. Actions vary from simple query evaluations or messaging commands to more complex behavior. The table for each protocol is accompanied by a figure, which illustrates how the protocol behaves within the context of the basic architecture introduced earlier in Figure 1. Figure 2. Protocol dimensions 3.1. The Direct Remote Query Protocol (DRQ) The Direct Remote Query Protocol (DRQ) is specified in Table 2 and depicted in Figure 3. Table 2 specifies how the protocol responds to a delta notification for relation R.3 A sym- metric protocol is used to handle updates to S. Protocols handling updates to R and to S may run concurrently. We will assume that multiple updates to R or to S are handled sequentially, although this assumption is not strictly necessary. Figure 3 illustrates the pro- tocol for both updates to R and updates to S. Note that arrows representing the transmission of query results are left out of the figure for reasons of clarity, as these arrows are implied by their counterparts representing queries. 

11 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 337 Table 2. DRQ Protocol 1 DBR send ∆R to CMR 2 CMR receive ∆R from DBR 3 send Q(∆R, S) to DBS 4 DBS receive Q(∆R, S) from CMR 5 evaluate Q(∆R, S) 6 send query result to CMR 7 CMR receive query result from DBS 8 raise alarm if query result is non-empty Figure 3. DRQ Protocol The DRQ protocol is very simple: When the constraint manager is notified of an update, it sends the appropriate incremental query for evaluation at the other site. Delta sets are usually small enough that the actual data can be transmitted in a straightforward way. For example, Q(∆R, S) may by a query over S with the values from ∆R “plugged in.” If the query result is non-empty, then the constraint manager raises an alarm. The DRQ protocol always detects when a constraint is violated, i.e., it is safe. Unfortunately, DRQ can easily produce “false alarms,” i.e., it is inaccurate. (As pointed out earlier, a protocol that raises an alarm every time there is an update also is safe but inaccurate. It should be evident that DRQ is much less inaccurate than such a protocol.) To show that the DRQ protocol is safe, we show that starting from a quiescent consistent global state D0 at time t0 , a quiescent inconsistent global state Dn at time tn cannot be reached without an alarm being raised: 1. At least one update must have occurred between times t0 and tn to reach an inconsistent quiescent state Dn from consistent state D0 . 2. Assume that update u1 occurring at time t1 , t0 < t1 < tn , is the last such update producing an inconsistent state. 3. Update u1 triggers a constraint checking process that does not raise an alarm, so there exists a time t2 , t1 < t2 < tn , at which the state is consistent. 

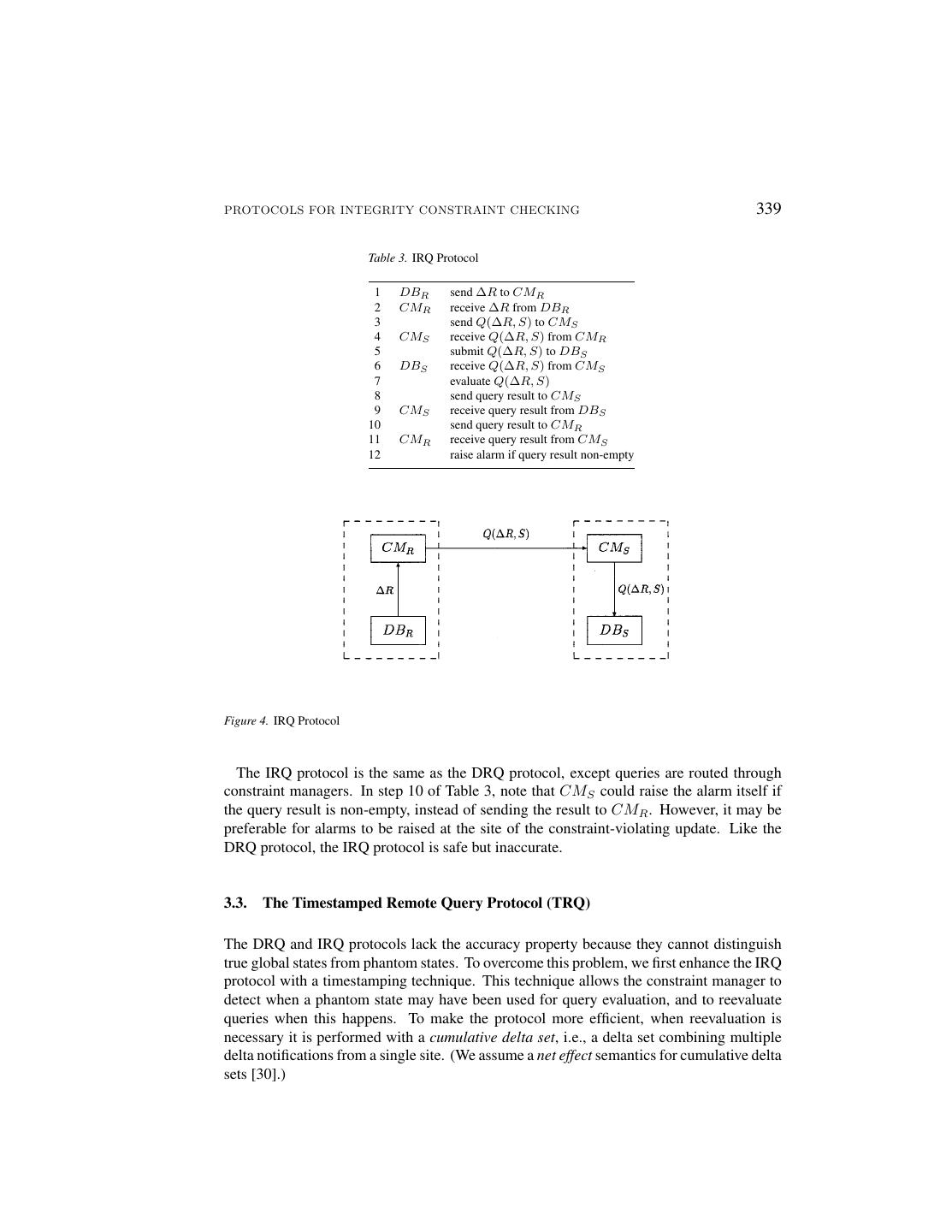
12 .338 GREFEN AND WIDOM 4. Since the state at tn is inconsistent, there must be an update u2 at time t3 , t2 < t3 < tn , that causes the inconsistent state. This contradicts the fact that u1 is the last update invalidating the constraint. The inaccuracy of DRQ is shown by a counterexample in which an alarm is raised but there is never an inconsistent global state. The counterexample is based on the example application from Section 2.5: 1. Consider constraint C1 , and let relations P atientsA and P atientsB both be empty in an initial (consistent) global database state at time t0 . 2. Suppose a local transaction is executed that inserts one tuple T with SSN = X into P atientsA and commits at time t1 , t0 < t1 . ∆P atientsA is sent to the constraint manager. 3. Let the triggered constraint checking process read relation P atientsB at time t2 , t1 < t2 . 4. Now suppose two other local transactions are executed, the first deleting tuple T from P atientsA and committing at time t3 , the second inserting a tuple T with SSN = X into P atientsB and committing at time t4 , such that t1 < t3 < t4 < t2 . 5. The DRQ protocol will raise an alarm after time t2 , even though no inconsistent state ever existed (since there was never more than one tuple in the database). Inaccuracy is due to the fact that the DRQ protocol may evaluate the constraint over a phantom state, since relations R and S are accessed at different times. Note again that the absence of global concurrency control mechanisms makes the traditional solution to this problem inapplicable here. We can solve the problem in two ways, by detection or by prevention: • We can use timestamping information to detect possible evaluation over phantom states. • We can exploit local transaction mechanisms to prevent evaluation over phantom states. The first solution is explored in Section 3.3, the second solution in Section 3.5. First, a slight variation on the DRQ protocol is presented. 3.2. The Indirect Remote Query Protocol (IRQ) The DRQ protocol relies on the capability of each database system to process queries issued from a remote constraint manager. If remote query services are unavailable, then we can use a variation on the DRQ protocol that uses peer-to-peer communication between the constraint managers. This Indirect Remote Query Protocol (IRQ) is specified in Table 3 and illustrated in Figure 4. For clarity, the figure (and all protocol figures to follow) only shows the case where R is updated. The case where S is updated is symmetric in all protocols. 

13 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 339 Table 3. IRQ Protocol 1 DBR send ∆R to CMR 2 CMR receive ∆R from DBR 3 send Q(∆R, S) to CMS 4 CMS receive Q(∆R, S) from CMR 5 submit Q(∆R, S) to DBS 6 DBS receive Q(∆R, S) from CMS 7 evaluate Q(∆R, S) 8 send query result to CMS 9 CMS receive query result from DBS 10 send query result to CMR 11 CMR receive query result from CMS 12 raise alarm if query result non-empty Figure 4. IRQ Protocol The IRQ protocol is the same as the DRQ protocol, except queries are routed through constraint managers. In step 10 of Table 3, note that CMS could raise the alarm itself if the query result is non-empty, instead of sending the result to CMR . However, it may be preferable for alarms to be raised at the site of the constraint-violating update. Like the DRQ protocol, the IRQ protocol is safe but inaccurate. 3.3. The Timestamped Remote Query Protocol (TRQ) The DRQ and IRQ protocols lack the accuracy property because they cannot distinguish true global states from phantom states. To overcome this problem, we first enhance the IRQ protocol with a timestamping technique. This technique allows the constraint manager to detect when a phantom state may have been used for query evaluation, and to reevaluate queries when this happens. To make the protocol more efficient, when reevaluation is necessary it is performed with a cumulative delta set, i.e., a delta set combining multiple delta notifications from a single site. (We assume a net effect semantics for cumulative delta sets [30].) 

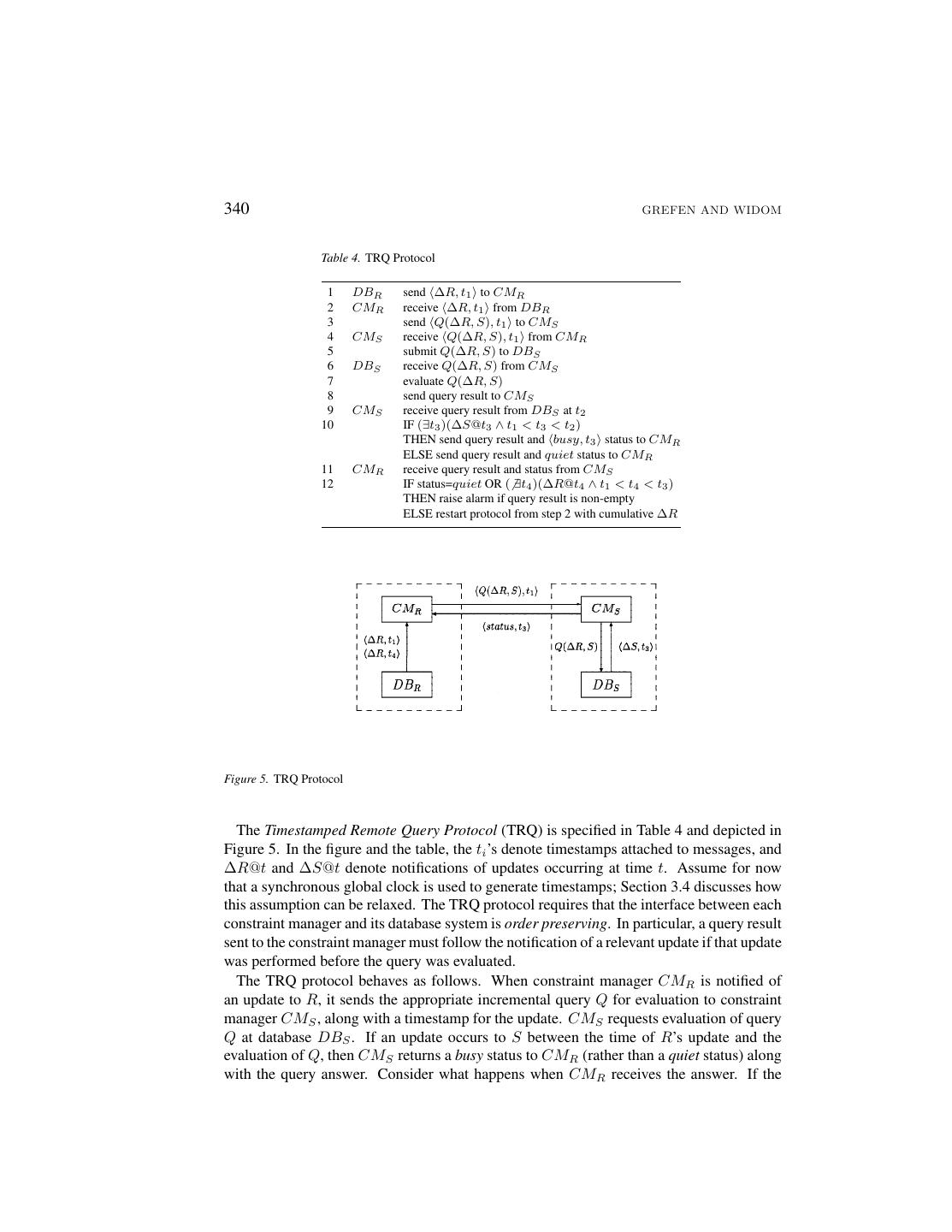
14 .340 GREFEN AND WIDOM Table 4. TRQ Protocol 1 DBR send ∆R, t1 to CMR 2 CMR receive ∆R, t1 from DBR 3 send Q(∆R, S), t1 to CMS 4 CMS receive Q(∆R, S), t1 from CMR 5 submit Q(∆R, S) to DBS 6 DBS receive Q(∆R, S) from CMS 7 evaluate Q(∆R, S) 8 send query result to CMS 9 CMS receive query result from DBS at t2 10 IF (∃t3 )(∆S@t3 ∧ t1 < t3 < t2 ) THEN send query result and busy, t3 status to CMR ELSE send query result and quiet status to CMR 11 CMR receive query result and status from CMS 12 IF status=quiet OR ( ∃t4 )(∆R@t4 ∧ t1 < t4 < t3 ) THEN raise alarm if query result is non-empty ELSE restart protocol from step 2 with cumulative ∆R Figure 5. TRQ Protocol The Timestamped Remote Query Protocol (TRQ) is specified in Table 4 and depicted in Figure 5. In the figure and the table, the ti ’s denote timestamps attached to messages, and ∆R@t and ∆S@t denote notifications of updates occurring at time t. Assume for now that a synchronous global clock is used to generate timestamps; Section 3.4 discusses how this assumption can be relaxed. The TRQ protocol requires that the interface between each constraint manager and its database system is order preserving. In particular, a query result sent to the constraint manager must follow the notification of a relevant update if that update was performed before the query was evaluated. The TRQ protocol behaves as follows. When constraint manager CMR is notified of an update to R, it sends the appropriate incremental query Q for evaluation to constraint manager CMS , along with a timestamp for the update. CMS requests evaluation of query Q at database DBS . If an update occurs to S between the time of R’s update and the evaluation of Q, then CMS returns a busy status to CMR (rather than a quiet status) along with the query answer. Consider what happens when CMR receives the answer. If the 

15 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 341 return status is quiet then there have been no relevant updates to S and the query has been evaluated over a true global state. If the return status is busy then there have been relevant updates to S. However, if R has not been updated further, then the query still has been evaluated over a true global state. In the case where there have been further updates to R, then the query may have been evaluated over a phantom state, and it must be reevaluated. Reevaluation takes place by restarting the protocol; for efficiency, the original and new updates to R are combined before restarting. Note that, provided that updates to R and S eventually cease, termination of the protocol is guaranteed since protocol restarts are triggered only by new updates. (In fact, updates at both sites are required to trigger a restart, so a given instance of the protocol is guaranteed to terminate as long as one site’s updates cease.) Safety of the TRQ protocol follows the same line of reasoning as safety for the DRQ protocol. We show that the TRQ protocol is accurate by arguing that every successful (i.e., not restarted) query evaluation is performed in a true global state. Hence, if an alarm is raised then there was a global state that violated the constraint: • After an update ∆R at time t1 , t1 is the first “candidate” global state for evaluation of Q. If evaluation does not produce a busy status, then Q has been evaluated in the state occurring at time t1 . • If CMS identifies a ∆S at time t3 with t1 < t3 < t2 , then t3 is taken as the next candidate global state. If there have been no further updates to R before t3 , then Q has been evaluated in the state occurring at time t3 . • If CMR identifies a new ∆R at time t4 with t1 < t4 < t3 , then the process is restarted with t3 as the next candidate global state. The TRQ protocol also can be used with time interval constraints. Time interval constraints require a constraint to be evaluated within a certain time interval, instead of on a single global database state. This type of constraint relaxes the usual notion of consistency of integrity constraints, but is sufficient for many environments (such as those with low update frequency). When the TRQ protocol is used for time interval constraints, the protocol is restarted in step 12 of Table 4 only if t3 − t4 is greater than the time interval specified for the constraint. 3.4. The Semi-Timestamped Remote Query Protocol (SRQ) The TRQ protocol assumes that the database systems can generate timestamps. A similar protocol can be used for the situation in which the database systems cannot generate times- tamps, but the constraint managers can.4 To provide the accuracy property when timestamps are generated by the constraint managers, it is necessary to guarantee that delta notifications from database systems are always received within a certain maximum delay t∆ [7]. For this scenario we provide the Semi-timestamped Remote Query Protocol (SRQ). SRQ is specified in Table 5 and depicted in Figure 6. In the figure, ∆R ↑ t∆ and ∆S ↑ t∆ indicate delta notifications within delay t∆ . The SRQ protocol is very similar to the TRQ protocol, 

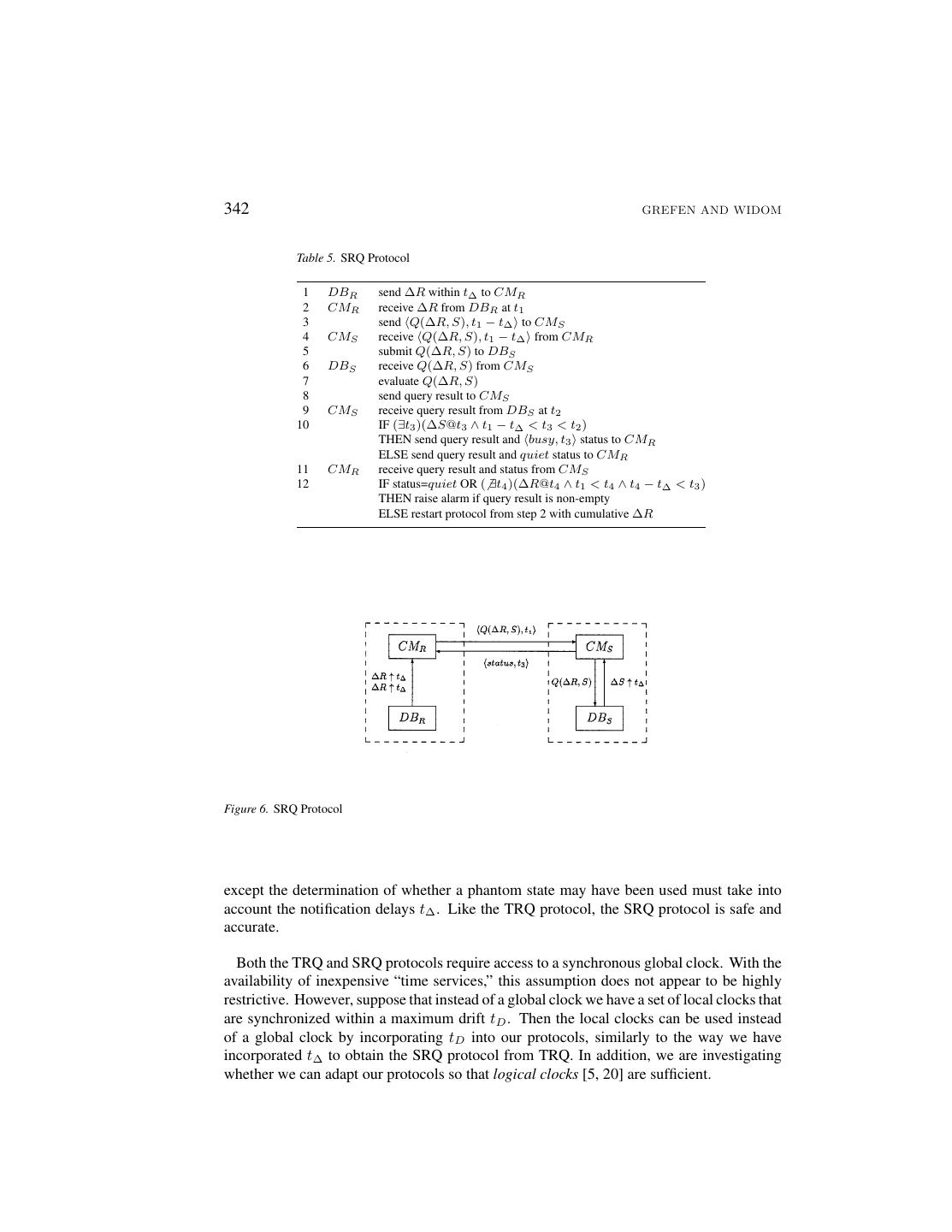
16 .342 GREFEN AND WIDOM Table 5. SRQ Protocol 1 DBR send ∆R within t∆ to CMR 2 CMR receive ∆R from DBR at t1 3 send Q(∆R, S), t1 − t∆ to CMS 4 CMS receive Q(∆R, S), t1 − t∆ from CMR 5 submit Q(∆R, S) to DBS 6 DBS receive Q(∆R, S) from CMS 7 evaluate Q(∆R, S) 8 send query result to CMS 9 CMS receive query result from DBS at t2 10 IF (∃t3 )(∆S@t3 ∧ t1 − t∆ < t3 < t2 ) THEN send query result and busy, t3 status to CMR ELSE send query result and quiet status to CMR 11 CMR receive query result and status from CMS 12 IF status=quiet OR ( ∃t4 )(∆R@t4 ∧ t1 < t4 ∧ t4 − t∆ < t3 ) THEN raise alarm if query result is non-empty ELSE restart protocol from step 2 with cumulative ∆R Figure 6. SRQ Protocol except the determination of whether a phantom state may have been used must take into account the notification delays t∆ . Like the TRQ protocol, the SRQ protocol is safe and accurate. Both the TRQ and SRQ protocols require access to a synchronous global clock. With the availability of inexpensive “time services,” this assumption does not appear to be highly restrictive. However, suppose that instead of a global clock we have a set of local clocks that are synchronized within a maximum drift tD . Then the local clocks can be used instead of a global clock by incorporating tD into our protocols, similarly to the way we have incorporated t∆ to obtain the SRQ protocol from TRQ. In addition, we are investigating whether we can adapt our protocols so that logical clocks [5, 20] are sufficient. 

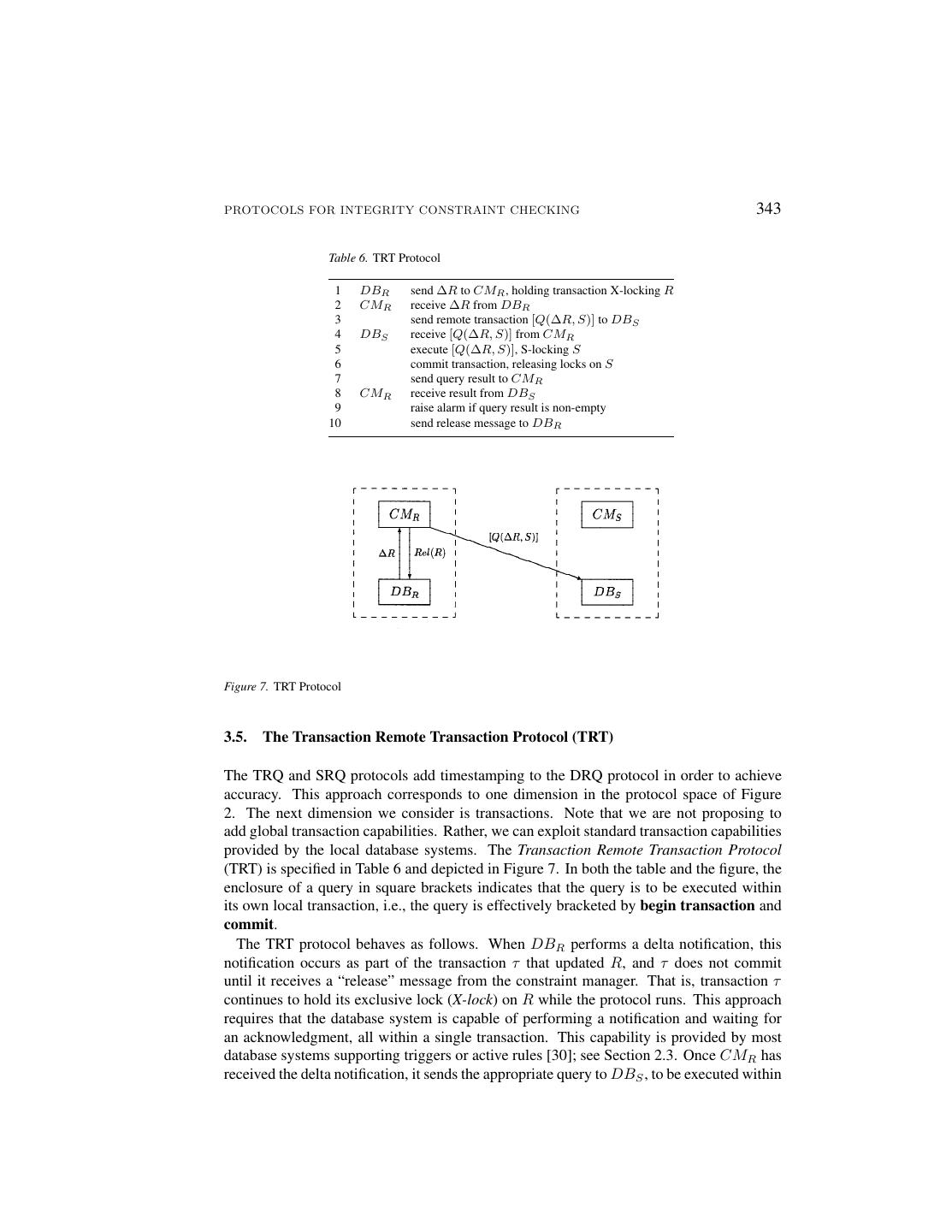
17 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 343 Table 6. TRT Protocol 1 DBR send ∆R to CMR , holding transaction X-locking R 2 CMR receive ∆R from DBR 3 send remote transaction [Q(∆R, S)] to DBS 4 DBS receive [Q(∆R, S)] from CMR 5 execute [Q(∆R, S)], S-locking S 6 commit transaction, releasing locks on S 7 send query result to CMR 8 CMR receive result from DBS 9 raise alarm if query result is non-empty 10 send release message to DBR Figure 7. TRT Protocol 3.5. The Transaction Remote Transaction Protocol (TRT) The TRQ and SRQ protocols add timestamping to the DRQ protocol in order to achieve accuracy. This approach corresponds to one dimension in the protocol space of Figure 2. The next dimension we consider is transactions. Note that we are not proposing to add global transaction capabilities. Rather, we can exploit standard transaction capabilities provided by the local database systems. The Transaction Remote Transaction Protocol (TRT) is specified in Table 6 and depicted in Figure 7. In both the table and the figure, the enclosure of a query in square brackets indicates that the query is to be executed within its own local transaction, i.e., the query is effectively bracketed by begin transaction and commit. The TRT protocol behaves as follows. When DBR performs a delta notification, this notification occurs as part of the transaction τ that updated R, and τ does not commit until it receives a “release” message from the constraint manager. That is, transaction τ continues to hold its exclusive lock (X-lock) on R while the protocol runs. This approach requires that the database system is capable of performing a notification and waiting for an acknowledgment, all within a single transaction. This capability is provided by most database systems supporting triggers or active rules [30]; see Section 2.3. Once CMR has received the delta notification, it sends the appropriate query to DBS , to be executed within 

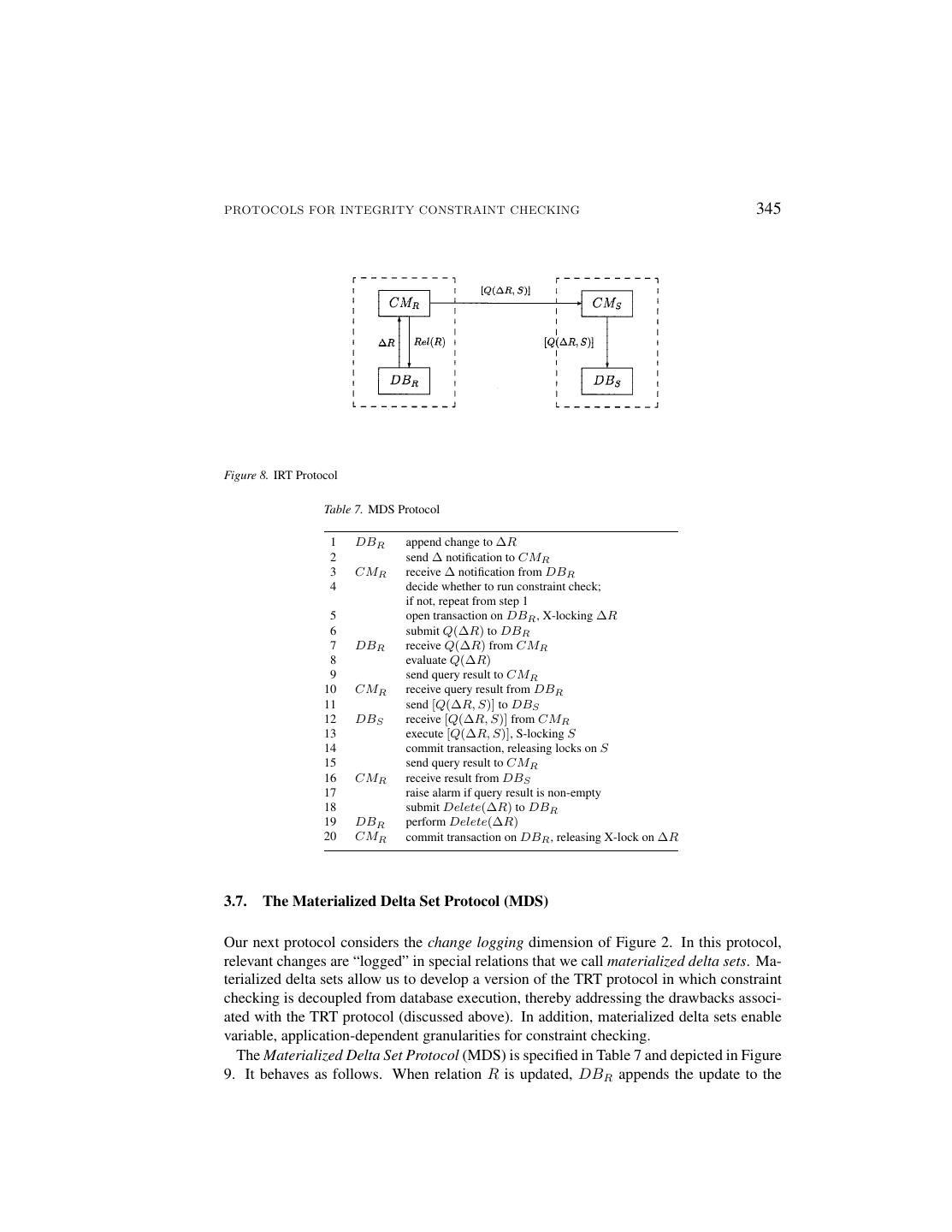
18 .344 GREFEN AND WIDOM its own transaction τ at DBS . During execution of the query, τ will hold a shared lock (S-lock) on S. When the query answer is received by CMR , an alarm is raised if the result is non-empty, and the pending transaction τ at DBR is released. Safety of the TRT protocol follows the same line of reasoning as for the other protocols. Accuracy of the TRT protocol results from the fact that every query is evaluated over a true global state (with respect to R and S). To see that only true global states are used, we can think of the bracketed query transaction τ as being embedded within the pending transaction τ that generated the delta notification. In this way, the TRT protocol effectively emulates a distributed two-phase locking protocol, ensuring serializability across sites with respect to R and S [23]. Note that although the TRT protocol emulates global locking, the protocol does not rely on global transaction mechanisms of any kind. Although the TRT protocol is relatively straightforward, and it satisfies both the safety and accuracy properties, it has two significant drawbacks: 1. Constraint manager processing is synchronous with the database system, unlike in the previous protocols. In TRT, the database system must wait for the constraint checking protocol to complete before it can commit its transaction and release its locks. This can be considered as a loss of autonomy, since progress on one site is dependent upon progress on the other site. 2. If R and S are updated concurrently then the risk of deadlock is relatively high. Since the TRT protocol emulates two-phase locking, the same deadlock cases arise as in central- ized or tightly-coupled distributed databases. However, executing a remote transaction in a loosely-coupled federated environment may be much slower than transaction pro- cessing in a tightly-coupled or centralized environment, thereby increasing the chance of deadlock. These drawbacks are addressed in an extended version of the TRT protocol called MDS, presented in Section 3.7. Another improvement to the TRT protocol is to use a local test to avoid the problems caused by remote transactions. This extension of the TRT protocol is described in Section 3.8. Below, we first present a slight variation on the TRT protocol. 3.6. The Indirect Transaction Remote Transaction Protocol (IRT) If direct submission of remote transactions is not supported by the database systems, an Indirect Transaction Remote Transaction Protocol (IRT) is possible. In this protocol, the bracketed query is sent to the remote database system through its constraint manager. IRT bears the same relationship to TRT that IRQ bears to DRQ (recall Section3.2). The protocol is depicted in Figure 8. Clearly, the IRT protocol has the same properties as the TRT protocol with respect to safety and accuracy. 

19 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 345 Figure 8. IRT Protocol Table 7. MDS Protocol 1 DBR append change to ∆R 2 send ∆ notification to CMR 3 CMR receive ∆ notification from DBR 4 decide whether to run constraint check; if not, repeat from step 1 5 open transaction on DBR , X-locking ∆R 6 submit Q(∆R) to DBR 7 DBR receive Q(∆R) from CMR 8 evaluate Q(∆R) 9 send query result to CMR 10 CMR receive query result from DBR 11 send [Q(∆R, S)] to DBS 12 DBS receive [Q(∆R, S)] from CMR 13 execute [Q(∆R, S)], S-locking S 14 commit transaction, releasing locks on S 15 send query result to CMR 16 CMR receive result from DBS 17 raise alarm if query result is non-empty 18 submit Delete(∆R) to DBR 19 DBR perform Delete(∆R) 20 CMR commit transaction on DBR , releasing X-lock on ∆R 3.7. The Materialized Delta Set Protocol (MDS) Our next protocol considers the change logging dimension of Figure 2. In this protocol, relevant changes are “logged” in special relations that we call materialized delta sets. Ma- terialized delta sets allow us to develop a version of the TRT protocol in which constraint checking is decoupled from database execution, thereby addressing the drawbacks associ- ated with the TRT protocol (discussed above). In addition, materialized delta sets enable variable, application-dependent granularities for constraint checking. The Materialized Delta Set Protocol (MDS) is specified in Table 7 and depicted in Figure 9. It behaves as follows. When relation R is updated, DBR appends the update to the 

20 .346 GREFEN AND WIDOM Figure 9. MDS Protocol materialized delta set ∆R. (Here, ∆R denotes an accumulated set of changes, not just an individual change or set of changes.) DBR ’s append to ∆R must occur before the local transaction that updates R commits; however, no other actions need occur within the updating transaction. A notification ∆ is sent to CMR , indicating that an update occurred but not providing the actual delta set. When CMR decides to proceed with constraint checking, it obtains an X-lock on ∆R for the duration of the protocol. CMR then reads ∆R, and proceeds just as in the TRT protocol, executing a query Q(∆R, S) within a transaction at the remote site. After receiving the query result and raising an alarm if appropriate, CMR erases the current contents of materialized delta set ∆R and releases its X-lock on ∆R. As in the TRT protocol, accuracy is guaranteed because the protocol emulates distributed two-phase locking. However, unlike in TRT, local access to R is not entirely restricted while the protocol is executing. Local transactions that read R can execute concurrently with the protocol. Concurrent local transactions also can update R; however, because the protocol X-locks ∆R, such transactions cannot complete and commit until the protocol has finished. This behavior still represents some loss of local autonomy, but it is a significant improvement over the TRT protocol. In addition, the risk of deadlock is much lower with MDS than with TRT, although deadlock is not eliminated altogether. A deadlock cycle can exist involving two update transactions, TU 1 and TU 2 at DBR and DBS respectively, and two constraint checking transactions, TC1 and TC2 at CMR and CMS respectively. The cycle is illustrated in Figure 10. Note that the occurrence of a four-element deadlock cycle is far less likely than the two-element cycle that can occur with the TRT protocol. 3.8. The Local Test Transaction Protocol (LTT) The last dimension of Figure 2 we explore is the use of local tests. Consider a delta notification ∆R. A local test is a query, QL (∆R, R), such that if the query result is empty, then the result of the global constraint checking query Q(∆R, S) is guaranteed to be empty as well. The test is “local” because it involves ∆R and R only, and does not require access to S. Hence, a local test can be used to avoid the many disadvantages of executing remote 

21 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 347 Figure 10. Deadlock in MDS protocol Table 8. LTT Protocol 1 DBR send ∆R to CMR , holding transaction X-locking R 2 CMR receive ∆R from DBR 3 submit QL (∆R, R) to DBR 4 DBR receive QL (∆R, R) from DBR 5 evaluate QL (∆R, R) 6 send query result to CMR 7 CMR receive query result from DBR 8 IF query result is non-empty THEN perform TRT protocol in same transaction Figure 11. LTT Protocol queries. Unfortunately, local tests are generally conservative, so if a local test fails it still becomes necessary to issue a remote query. A considerable theory of local tests has been developed in [18, 17]. Here we show how that theory can be put into practice in the context of constraint checking protocols for federated databases. As an example of a constraint with a local test, recall factored constraint C3B from Sec- tion 2.5: C3B : ΠP hysician ∆+ P atientsB − ΠP hN P hysicians 

22 .348 GREFEN AND WIDOM This constraint specifies that new patient records must match existing physician records. If all new patient records match physicians in existing patient records, then this local condition is sufficient to guarantee validity of the constraint without examining the physician records (under the assumption that the existing patient records satisfy the constraint). The local test is expressed as the following query, where the test succeeds if the query result is empty: C3BL : ΠP hysician ∆+ P atientsB − ΠP hysician ∆0 P atientsB The Local Test Transaction Protocol (LTT) is specified in Table 8 and depicted in Figure 11. The protocol is very similar to TRT, except before issuing the remote transaction Q(∆R, S), the local test QL (∆R, R) is evaluated. If the local test succeeds, then the protocol terminates successfully with no remote activity. Note that the local test must be evaluated within the same transaction in which the update occurred in order to behave correctly [17, 31], so a materialized delta set approach cannot be applied here. The safety and accuracy of the LTT protocol follows from the correctness of local tests [18, 17], and from the safety and accuracy of the TRT protocol. 4. Analyzing the Family of Protocols In Section 3 we developed a family of constraint checking protocols along the four dimen- sions introduced in Figure 2. In this section we analyze the protocols. First, we consider the protocols in the context of the four-dimensional space to see how complete the family is. Then we compare the characteristics and costs of the various protocols. This comparison yields a “consumer report” that can be used to select the most appropriate protocol for a specific federated database environment. Finally, we discuss how the protocols can be extended to handle constraints spanning more than two databases. 4.1. The Protocol Space Figure 12 places the protocols developed in Section 3 into their appropriate positions in the four-dimensional space of Figure 2. Although several combinations of dimensions have been explored, not every possibility has been considered. The four dimensions yield sixteen different possible protocols, listed in Table 9. We first point out three potentially useful protocols not discussed in Section 3. These protocols combine features from our protocols in a relatively straightforward way. 1. Change logging can be used with the DRQ or IRQ protocol to produce a Cumulative Remote Query (CRQ) protocol. CRQ accumulates changes in a materialized delta set to allow variable granularities of constraint checking. When a remote query is posed, it uses the current contents of the materialized delta set. 2. Timestamps and change logging can be combined to obtain a Timestamped Materi- alized Delta Set (TMD) protocol. In this protocol, materialized delta sets containing timestamps are used in order to allow variable granularities of constraint checking and to keep track of cumulative updates for protocol restarts. 

23 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 349 Figure 12. Protocols in the protocol space Table 9. Protocol space filled in timestamps transactions change logging local tests Protocol 1 DRQ, IRQ 2 X TRQ, SRQ 3 X TRT, IRT 4 X X inappropriate 5 X CRQ (see text) 6 X X TMD (see text) 7 X X MDS 8 X X X inappropriate 9 X inappropriate 10 X X inappropriate 11 X X LTT 12 X X X LTG (see text) 13 X X inappropriate 14 X X X inappropriate 15 X X X to be considered 16 X X X X to be considered 3. Timestamps, transaction mechanisms, and local tests can be combined to obtain a Local Transaction Global Timestamped (LTG) protocol. The LTG protocol resembles the LTT protocol, except when a local test fails, timestamping is used rather than transactions for the global query (to achieve more autonomy). Some protocols appearing in Table 9 have an inappropriate combination of features: Local tests cannot be used without local transactions. (Otherwise, the query for the local test might be evaluated in an erroneous state [18, 31].) This eliminates lines 9–10 and 13–14. Combining timestamps and transactions is “overkill” in the case where local tests are not used (eliminating lines 4 and 8). Finally, the combination of transactions, change logging, 

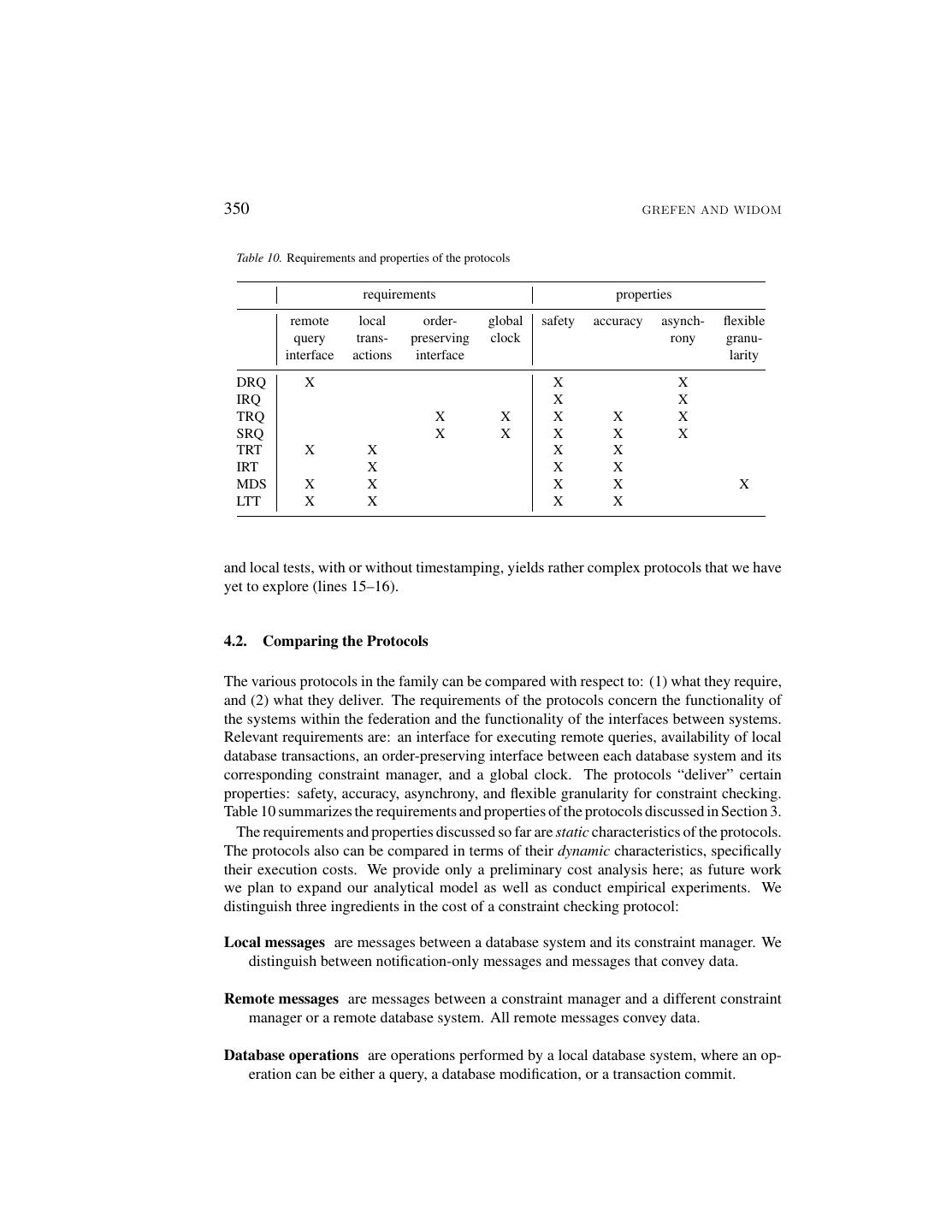
24 .350 GREFEN AND WIDOM Table 10. Requirements and properties of the protocols requirements properties remote local order- global safety accuracy asynch- flexible query trans- preserving clock rony granu- interface actions interface larity DRQ X X X IRQ X X TRQ X X X X X SRQ X X X X X TRT X X X X IRT X X X MDS X X X X X LTT X X X X and local tests, with or without timestamping, yields rather complex protocols that we have yet to explore (lines 15–16). 4.2. Comparing the Protocols The various protocols in the family can be compared with respect to: (1) what they require, and (2) what they deliver. The requirements of the protocols concern the functionality of the systems within the federation and the functionality of the interfaces between systems. Relevant requirements are: an interface for executing remote queries, availability of local database transactions, an order-preserving interface between each database system and its corresponding constraint manager, and a global clock. The protocols “deliver” certain properties: safety, accuracy, asynchrony, and flexible granularity for constraint checking. Table 10 summarizes the requirements and properties of the protocols discussed in Section 3. The requirements and properties discussed so far are static characteristics of the protocols. The protocols also can be compared in terms of their dynamic characteristics, specifically their execution costs. We provide only a preliminary cost analysis here; as future work we plan to expand our analytical model as well as conduct empirical experiments. We distinguish three ingredients in the cost of a constraint checking protocol: Local messages are messages between a database system and its constraint manager. We distinguish between notification-only messages and messages that convey data. Remote messages are messages between a constraint manager and a different constraint manager or a remote database system. All remote messages convey data. Database operations are operations performed by a local database system, where an op- eration can be either a query, a database modification, or a transaction commit. 

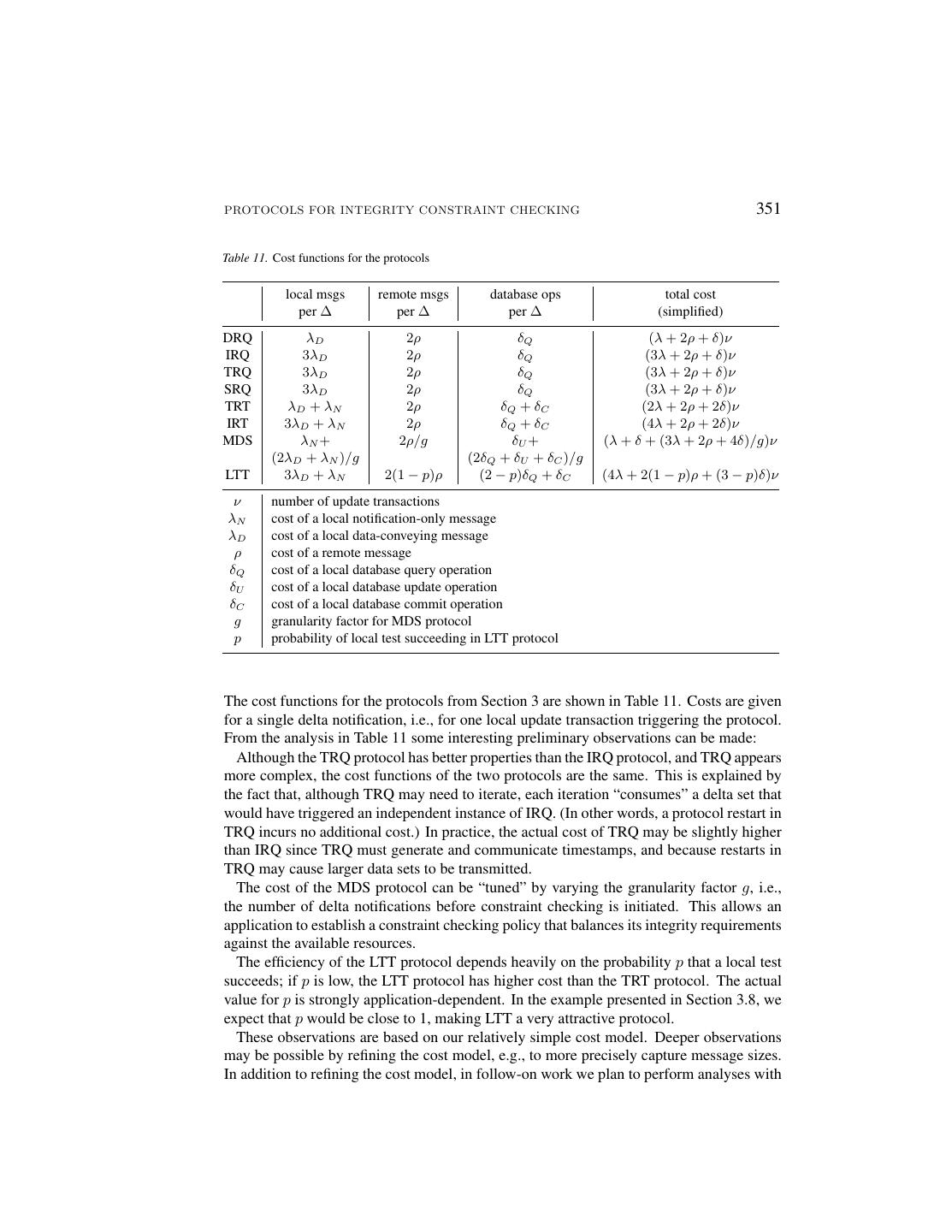
25 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 351 Table 11. Cost functions for the protocols local msgs remote msgs database ops total cost per ∆ per ∆ per ∆ (simplified) DRQ λD 2ρ δQ (λ + 2ρ + δ)ν IRQ 3λD 2ρ δQ (3λ + 2ρ + δ)ν TRQ 3λD 2ρ δQ (3λ + 2ρ + δ)ν SRQ 3λD 2ρ δQ (3λ + 2ρ + δ)ν TRT λD + λN 2ρ δQ + δC (2λ + 2ρ + 2δ)ν IRT 3λD + λN 2ρ δQ + δC (4λ + 2ρ + 2δ)ν MDS λN + 2ρ/g δU + (λ + δ + (3λ + 2ρ + 4δ)/g)ν (2λD + λN )/g (2δQ + δU + δC )/g LTT 3λD + λN 2(1 − p)ρ (2 − p)δQ + δC (4λ + 2(1 − p)ρ + (3 − p)δ)ν ν number of update transactions λN cost of a local notification-only message λD cost of a local data-conveying message ρ cost of a remote message δQ cost of a local database query operation δU cost of a local database update operation δC cost of a local database commit operation g granularity factor for MDS protocol p probability of local test succeeding in LTT protocol The cost functions for the protocols from Section 3 are shown in Table 11. Costs are given for a single delta notification, i.e., for one local update transaction triggering the protocol. From the analysis in Table 11 some interesting preliminary observations can be made: Although the TRQ protocol has better properties than the IRQ protocol, and TRQ appears more complex, the cost functions of the two protocols are the same. This is explained by the fact that, although TRQ may need to iterate, each iteration “consumes” a delta set that would have triggered an independent instance of IRQ. (In other words, a protocol restart in TRQ incurs no additional cost.) In practice, the actual cost of TRQ may be slightly higher than IRQ since TRQ must generate and communicate timestamps, and because restarts in TRQ may cause larger data sets to be transmitted. The cost of the MDS protocol can be “tuned” by varying the granularity factor g, i.e., the number of delta notifications before constraint checking is initiated. This allows an application to establish a constraint checking policy that balances its integrity requirements against the available resources. The efficiency of the LTT protocol depends heavily on the probability p that a local test succeeds; if p is low, the LTT protocol has higher cost than the TRT protocol. The actual value for p is strongly application-dependent. In the example presented in Section 3.8, we expect that p would be close to 1, making LTT a very attractive protocol. These observations are based on our relatively simple cost model. Deeper observations may be possible by refining the cost model, e.g., to more precisely capture message sizes. In addition to refining the cost model, in follow-on work we plan to perform analyses with 

26 .352 GREFEN AND WIDOM varying parameter values in order to further understand the relative costs of the protocols in various environments. 4.3. Multi-Site Constraints Our constraint checking protocols in Section 3 have been developed for constraints involving exactly two databases. It is our experience that two-site constraints predominate in practice. Furthermore, even when a constraint involves more than two databases, often it can be “split” into multiple constraints, each involving exactly two databases. For example, suppose our hospital application (Section 2.5) involved three hospitals instead of two. The constraint specifying that each patient can be registered at only one hospital (constraint C1 ) now involves three sites. However, instead of enforcing the three-site constraint directly, we can enforce the following set of two-site constraints: C11 : P atientsA ✶SSN =SSN P atientsB C12 : P atientsB ✶SSN =SSN P atientsC C13 : P atientsA ✶SSN =SSN P atientsC Together, these constraints imply the desired three-site constraint. There are, however, cases where we may need to handle multi-site constraints: We may wish to handle constraints such as the example above directly, rather than separately handling a set of equivalent constraints (which may be exponential in number). Furthermore, some constraints cannot be split as illustrated above. Suppose in our three-hospital example that patients may register at two hospitals but not three. On insertions into relation P atientsA , the constraint checking protocol must evaluate the following incremental query: ΠSSN (P atientsA+ ✶SSN =SSN P atientsB) ∩ ΠSSN (P atientsA+ ✶SSN =SSN P atientsC) When a delta notification for P atientsA is received by the constraint manager at site SA , this query can be evaluated easily using the DRQ or IRQ protocol—the constraint manager simply submits two remote queries (one to SB and one to SC ) and intersects the results. Safety of the protocol is guaranteed by the same reasoning given in Section 3.1. Timestamp- based protocols TRQ and SRQ also can be extended in a straightforward way to handle multi-site constraints: A restart is necessary whenever a busy status is received from any of the remote sites and a local update has occurred; safety and accuracy follow from the results in Section 3.3. It is less straightforward to construct safe and accurate versions of the transaction-based protocols (such as TRT) for the multi-site case. These protocols emulate two-phase locking, which requires coordinating local transactions across multiple sites. A two-phase “hand- shake” protocol could be used for synchronization, similar to distributed two-phase commit. Alternatively, a mechanism could be used where remote queries are “chained” from one site to the next (emulating multiple nested transactions). Unfortunately, both solutions incur a considerable loss of local autonomy. 

27 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 353 These initial observations lead us to believe that non-transaction-based protocols are most suitable for constraints involving more than two sites. As future work we plan to explore the issues more carefully, and to develop protocols especially suited to the multi-site case. 5. Conclusions and Future Work We have described a family of constraint checking protocols for federated database systems. The protocols have been developed by starting with a straightforward basic protocol, then improving upon the basic protocol by extending it along a number of dimensions. We have isolated two properties of constraint checking protocols that are of primary importance: safety and accuracy. All of our protocols are safe, meaning that they are guaranteed to detect every constraint violation. A protocol is accurate if it does not raise “false alarms,” i.e., it only detects true constraint violations. Obtaining accuracy requires additional mechanisms, as seen in our more complex protocols. In addition to varying in terms of accuracy, our protocols also vary in terms of their requirements of the underlying systems, their level of asynchrony, their flexibility, and their execution costs. We are quite certain that no one protocol will be suitable for all federated database scenarios. By providing a family of alternatives, one of our protocols can be chosen—and perhaps tailored—for a particular environment or application. By formalizing the relevant concepts, and by identifying and analyzing a suite of protocols, this paper provides a sound basis for the problem of integrity constraint checking in federated databases. The work presented in this paper can be extended in a number of directions: • The model for analyzing the execution costs of the protocols can be further elaborated. So far we have identified the cost “ingredients,” and we have compared the protocols according to these ingredients. The next steps are to introduce and vary parameter values to understand relative costs in different environments, and to drop some of the simplifying assumptions we made for the initial cost analysis. • The issue of constraint repair when violations occur requires further study. Even in traditional centralized databases, constraint repair is an important topic of current research (e.g., [6, 15]). In federated databases the problem is even more difficult. It has to be determined which of the protocols can be extended to incorporate constraint repair, and what the characteristics of these extended protocols are with respect to constraint maintenance properties, system requirements, and execution costs. • The protocols identified by Table 9 that we have not studied but that may be applicable for certain environments merit further study. The same holds for protocols for multi- site constraints (expanding on the ideas of Section 4.3), and for protocols for handling constraints that do not have a straightforward incremental form. • The use of logical clocks [5, 20] instead of absolute timestamps is an interesting mod- ification to be studied for those protocols that currently rely on synchronized time services. 

28 .354 GREFEN AND WIDOM Acknowledgments We are grateful to Stefano Ceri, Hector Garcia-Molina, and the rest of the Stanford Database Group for useful feedback on this work. Notes 1. In this paper we do not consider the reaction to constraint violations, although it is an important area of future work. Rather, we focus on the detection of constraint violations, and we say that when a protocol detects a constraint violation it raises an “alarm.” 2. Here a global clock may be required; see Section 3.3. 3. Recall that a delta notification is associated with a delta set ∆R. The delta set may contain the updates performed by a local transaction, or it may reflect some other unit of work relevant for local database DBR . 4. As a general principle, we assume that the capabilities of the local database systems are likely to be fixed in advance (e.g., in the case of legacy systems), while constraint managers may permit modifications and enhancements for the purposes of implementing the protocols. References 1. D. Bell and J. Grimson. Distributed Database Systems. Addison-Wesley, Reading, Massachusetts, USA, 1992. 2. D. Barbara and H. Garcia-Molina. The Demarcation Protocol: A technique for maintaining linear arithmetic constraints in distributed database systems. In Advances in Database Technology—EDBT ’92, Lecture Notes in Computer Science 580. Springer-Verlag, Berlin, 1992. 3. Y. Breitbart, H. Garcia-Molina, and A. Silberschatz. Overview of multidatabase transaction management. VLDB Journal, 1(2), 1992. 4. J.A. Blakeley, P.-A. Larson, and F.W. Tompa. Efficiently updating materialized views. In Procs. ACM SIGMOD Int. Conf. on Management of Data, Washington, D.C., 1986. 5. O. Babao˘ glu and K. Marzullo. Consistent global states of distributed systems: Fundamental concepts and mechanisms. In S. Mullender, editor, Distributed Systems. ACM Press, New York, 1993. 6. S. Ceri, P. Fraternali, S. Paraboschi, and L. Tanca. Automatic generation of production rules for integrity maintenance. ACM Trans. on Database Systems, 19(3), 1994. 7. S. Chawathe, H. Garcia-Molina, and J. Widom. A Toolkit for Constraint Management in Heterogeneous Information Systems. In Procs. 12th Int. Conf. on Data Engineering, New Orleans, LA, 1996. 8. K.M. Chandy and L. Lamport. Distributed snapshots: Determining global states of distributed systems. ACM Trans. on Computer Systems, 3(1), 1985. 9. S. Ceri and J. Widom. Managing semantic heterogeneity with production rules and persistent queues. In Procs. 19th Int. Conf. on Very Large Data Bases, Dublin, Ireland, 1993. 10. L. Do and P. Drew. Active database management of global data integrity constraints in heterogeneous database environments. In Procs. 11th Int. Conf. on Data Engineering, Taipei, Taiwan, 1995. 11. A. Elmagarmid (ed.). Special Issue on Unconventional Transaction Management, Data Engineering Bulletin 14(1), 1991. 12. A. Elmagarmid (ed.). Extended Transaction Models for Advanced Applications. Morgan Kaufmann, San Mateo, California, USA, 1992. 13. P.W.P.J. Grefen and P.M.G. Apers. Parallel handling of integrity constraints on fragmented relations. In Procs. Int. Symp. on Databases in Parallel and Distributed Systems, Dublin, Ireland, 1990. 14. P.W.P.J. Grefen and P.M.G. Apers. Integrity control in relational database systems – an overview. Journal of Data & Knowledge Engineering, 10(2), 1993. 15. M. Gertz and U.W. Lipeck. Deriving integrity maintaining triggers from transition graphs. In Procs. 9th Int. Conf. on Data Engineering, Vienna, Austria, 1993. 

29 .PROTOCOLS FOR INTEGRITY CONSTRAINT CHECKING 355 16. P.W.P.J. Grefen. Combining theory and practice in integrity control: a declarative approach to the specification of a transaction modification subsystem. In Procs. 19th Int. Conf. on Very Large Data Bases, Dublin, Ireland, 1993. 17. A. Gupta, Y. Sagiv, J.D. Ullman, and J. Widom. Constraint checking with partial information. In Procs. 13th ACM SIGACT-SIGMOD-SIGART Symp. on Principles of Database Systems, 1994. 18. A. Gupta and J. Widom. Local verification of global integrity constraints in distributed databases. In Procs. ACM SIGMOD Int. Conf. on Management of Data, Washington, D.C., 1993. 19. P. Grefen and J. Widom. Integrity constraint checking in federated databases. Memoranda Informatica, 94-80, Department of Computer Science, University of Twente, The Netherlands, 1994. 20. L. Lamport. Time, clocks, and the ordering of events in a distributed system. Communications ACM, 21(7), 1978. 21. D. Montesi and R. Torlone. A transaction transformation approach to active rule processing. In Procs. 11th Int. Conf. on Data Engineering, Taipei, Taiwan, 1995. 22. J.-M. Nicolas. Logic for improving integrity checking in relational data bases. Acta Informatica, 18, 1982. 23. T. Oszu and P. Valduriez. Principles of Distributed Database Systems. Prentice-Hall, Englewood Cliffs, New Jersey, 1991. 24. X. Qian. Distribution design of integrity constraints. In L. Kerschberg, editor, Expert Database Systems— Procs. from the 2nd Int. Conf.. Benjamin/Cummings, Redwood City, California, 1989. 25. X. Qian and G. Wiederhold. Incremental recomputation of active relational expressions. IEEE Trans. on Knowledge and Data Engineering, 3(3), 1991. 26. A. Rosenthal, S. Chakravarthy, B. Blaustein, and J. Blakeley. Situation monitoring for active databases. In Procs. 15th Int. Conf. on Very Large Data Bases, Amsterdam, The Netherlands, 1989. 27. M. Rusinkiewicz, A. Sheth, and G. Karabatis. Specifying interdatabase dependencies in a multidatabase environment. IEEE Computer, 24(12), 1991. 28. E. Simon and P. Valduriez. Integrity control in distributed database systems. In Procs. 19th Int. Conf. on System Sciences, Hawaii, 1986. 29. J.D. Ullman. Principles of Database and Knowledge-Base Systems, Volumes I and II. Computer Science Press, Rockville, Maryland, 1989. 30. J. Widom and S. Ceri. Active Database Systems: Triggers and Rules for Advanced Database Processing. Morgan Kaufmann, San Francisco, California, 1996. 31. Y. Zhuge, H. Garcia-Molina, J. Hammer, and J. Widom. View maintenance in a warehousing environment. In Procs. ACM SIGMOD Int. Conf. on Management of Data, San Jose, California, 1995.