展开查看详情
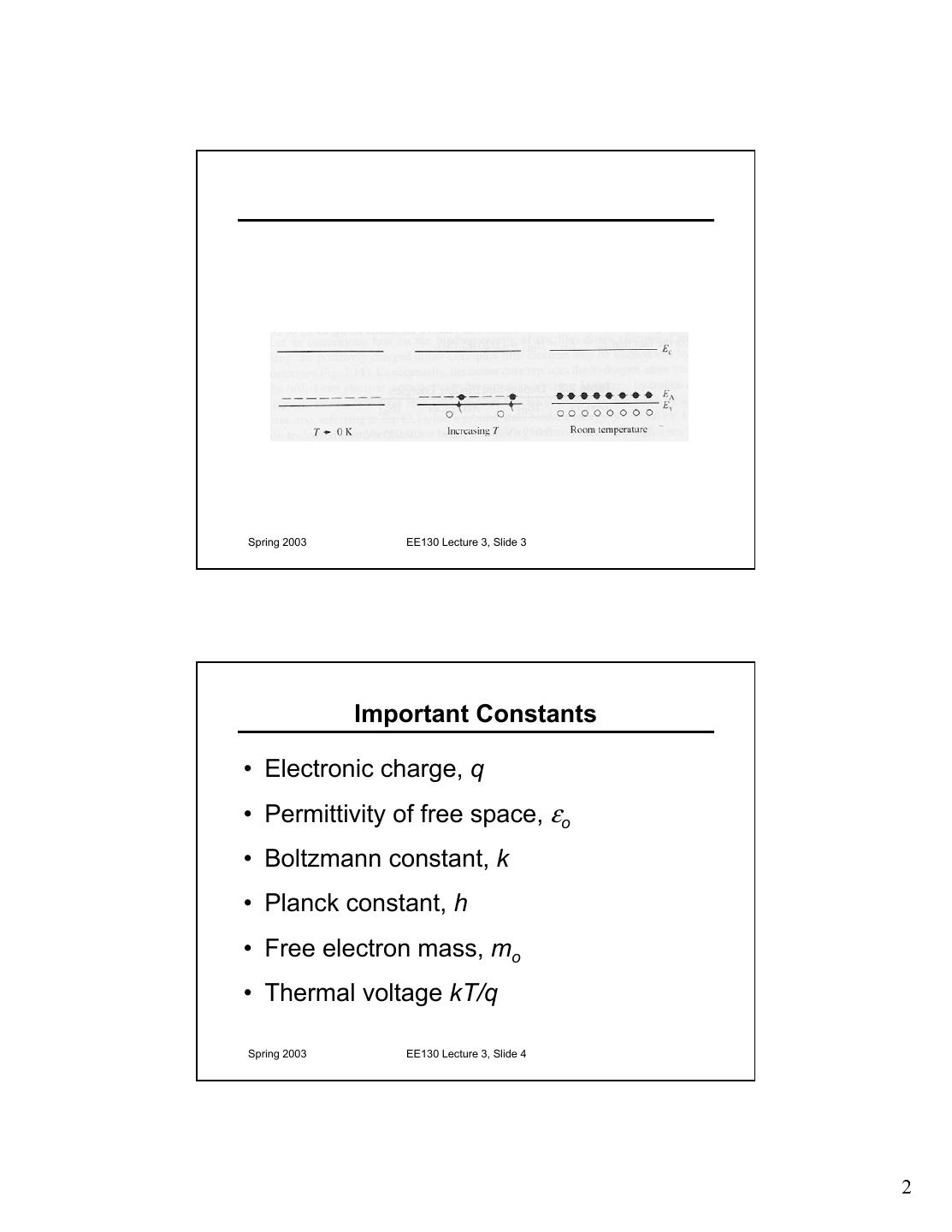
1 . Lecture #3
OUTLINE
• Thermal equilibrium
• Fermi-Dirac distribution
– Boltzmann approximation
• Relationship between EF and n, p
• Temperature dependence of EF, n, p
Finish reading Chapter 2
Spring 2003 EE130 Lecture 3, Slide 1
Review: Energy Band Model and Doping
Spring 2003 EE130 Lecture 3, Slide 2
1
2 .Spring 2003 EE130 Lecture 3, Slide 3
Important Constants
• Electronic charge, q
• Permittivity of free space, εo
• Boltzmann constant, k
• Planck constant, h
• Free electron mass, mo
• Thermal voltage kT/q
Spring 2003 EE130 Lecture 3, Slide 4
2
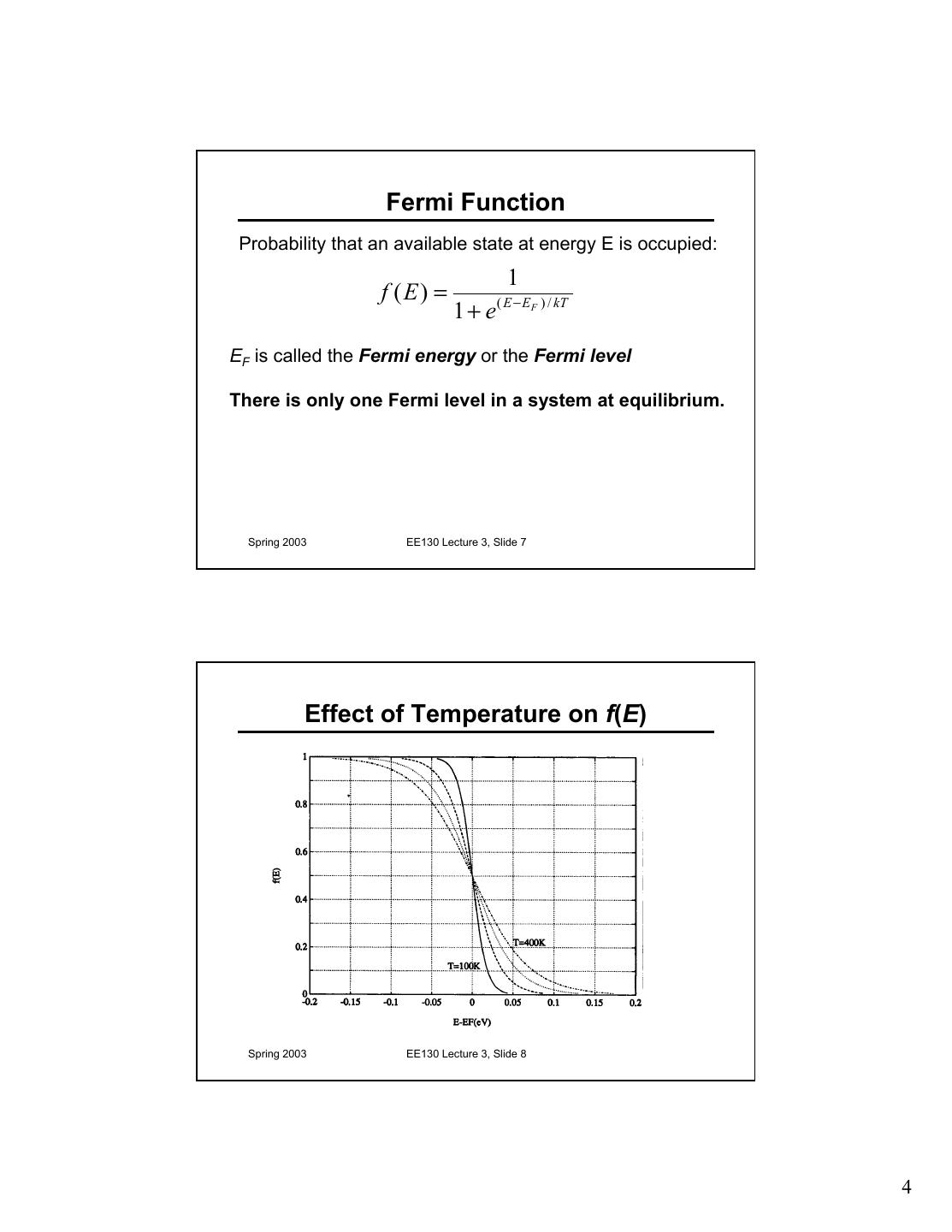
3 . Thermal Equilibrium
• No external forces applied:
– electric field = 0
– magnetic field = 0
– mechanical stress = 0
• Thermal agitation –> electrons and holes exchange
energy with the crystal lattice and each other
⇒ Every energy state in the conduction and valence
bands has a certain probability of being occupied by an
electron
Spring 2003 EE130 Lecture 3, Slide 5
Analogy for Thermal Equilibrium
Sand particles
Dish
Vibrating table
• There is a certain probability for the electrons in the
conduction band to occupy high-energy states under
the agitation of thermal energy (vibrating atoms, etc.)
Spring 2003 EE130 Lecture 3, Slide 6
3
4 . Fermi Function
Probability that an available state at energy E is occupied:
1
f (E) =
1 + e( E − EF ) / kT
EF is called the Fermi energy or the Fermi level
There is only one Fermi level in a system at equilibrium.
Spring 2003 EE130 Lecture 3, Slide 7
Effect of Temperature on f(E)
Spring 2003 EE130 Lecture 3, Slide 8
4
5 . Boltzmann Approximation
If E − E F > 3kT , f ( E ) ≈ e − ( E − EF ) kT
If E F − E > 3kT , f ( E ) ≈ 1 − e E − EF kT
Spring 2003 EE130 Lecture 3, Slide 9
Density of States
E
gc(E)
∆Ε
Ec Ec
Ev Ev
gv(E)
g(E)dE = number of states per cm3 in the energy range between E and E+dE
Near the band edges:
mn* 2mn* (E − Ec )
gc ( E ) = E ≥ Ec
π 2h 3
m*p 2m*p (Ev − E )
gv ( E ) = E ≤ Ev
π 2h 3
Spring 2003 EE130 Lecture 3, Slide 10
5
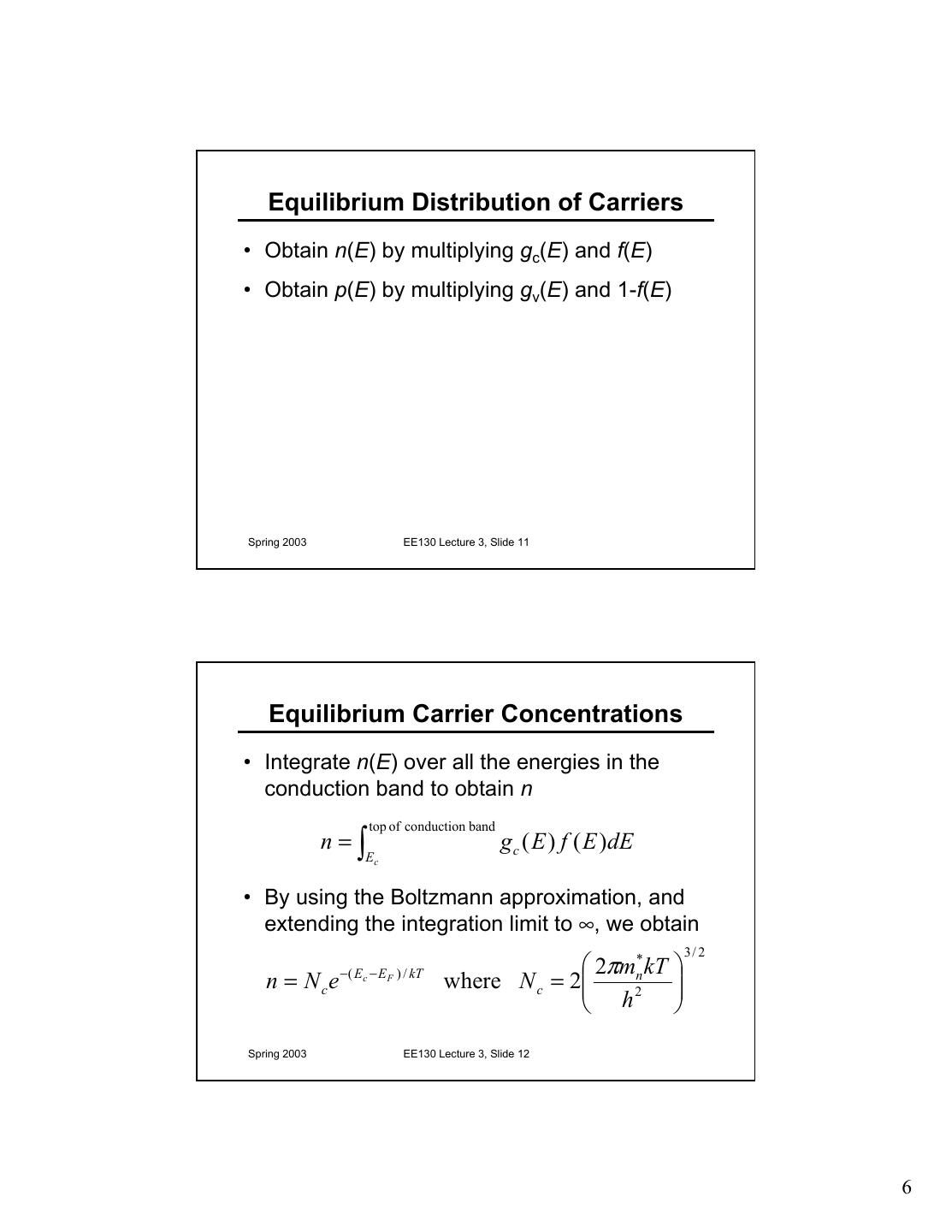
6 . Equilibrium Distribution of Carriers
• Obtain n(E) by multiplying gc(E) and f(E)
• Obtain p(E) by multiplying gv(E) and 1-f(E)
Spring 2003 EE130 Lecture 3, Slide 11
Equilibrium Carrier Concentrations
• Integrate n(E) over all the energies in the
conduction band to obtain n
top of conduction band
n=∫ g c ( E ) f ( E )dE
Ec
• By using the Boltzmann approximation, and
extending the integration limit to ∞, we obtain
3/ 2
2πmn*kT
n = N c e −( Ec − EF ) / kT where N c = 2 2
h
Spring 2003 EE130 Lecture 3, Slide 12
6
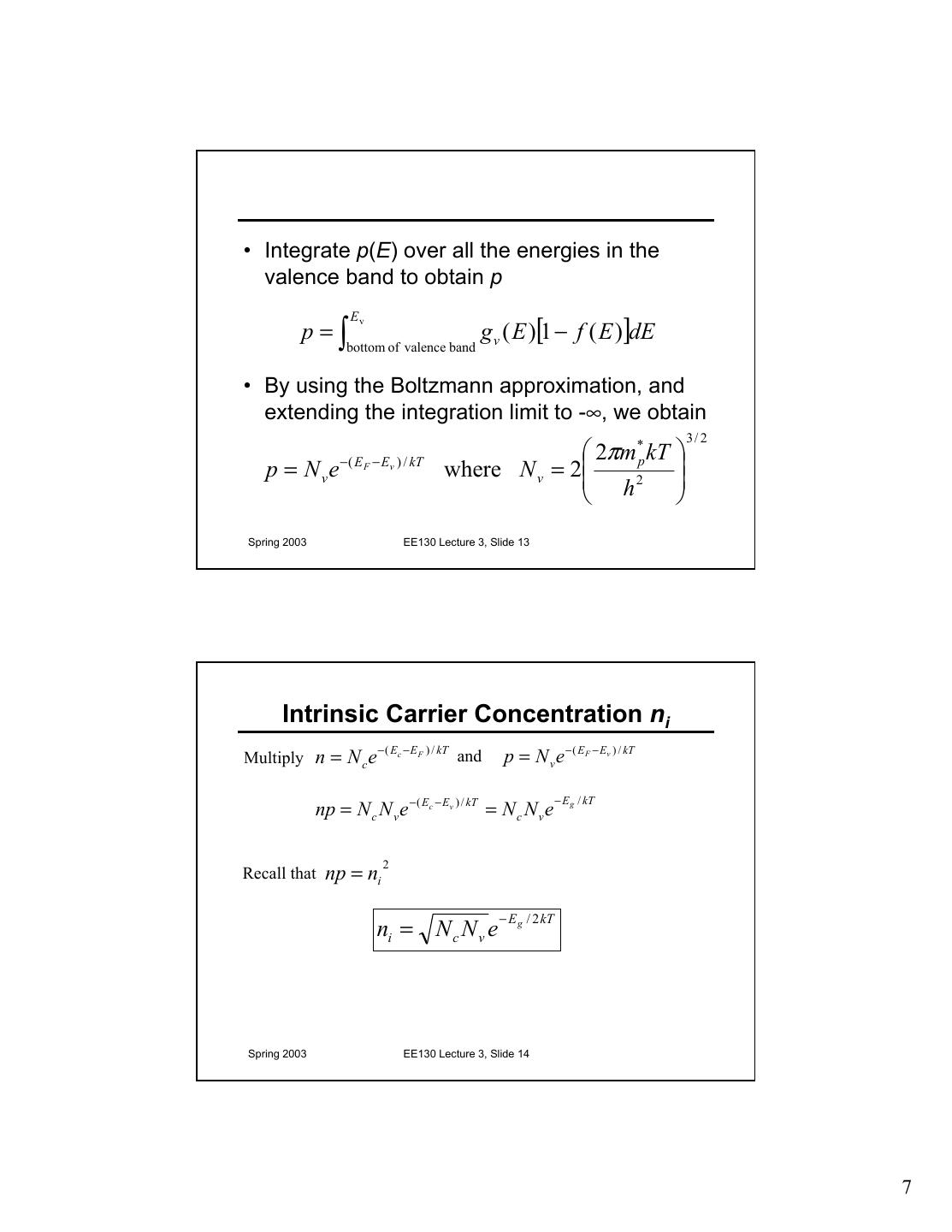
7 .• Integrate p(E) over all the energies in the
valence band to obtain p
g v ( E )[1 − f ( E )]dE
Ev
p=∫
bottom of valence band
• By using the Boltzmann approximation, and
extending the integration limit to -∞, we obtain
3/ 2
2πm*p kT
p = N ve − ( E F − Ev ) / kT
where N v = 2 2
h
Spring 2003 EE130 Lecture 3, Slide 13
Intrinsic Carrier Concentration ni
Multiply n = N c e
− ( Ec − E F ) / kT
and p = N v e − ( EF − Ev ) / kT
− E g / kT
np = N c N v e − ( Ec − Ev ) / kT = N c N v e
2
Recall that np = ni
− E g / 2 kT
ni = N c N v e
Spring 2003 EE130 Lecture 3, Slide 14
7
8 . Intrinsic Fermi Level
• To find EF for an intrinsic semiconductor (n = p = ni):
n = N c e( Ei − EC ) / kT = N v e( EV − Ei ) / kT = p
EC + EV kT NV
Ei = + ln
2 2 N C
EC + EV 3kT m p
*
Ei = + ln *
2 4 mn
Spring 2003 EE130 Lecture 3, Slide 15
n(ni, EF) and p(ni, EF)
Spring 2003 EE130 Lecture 3, Slide 16
8
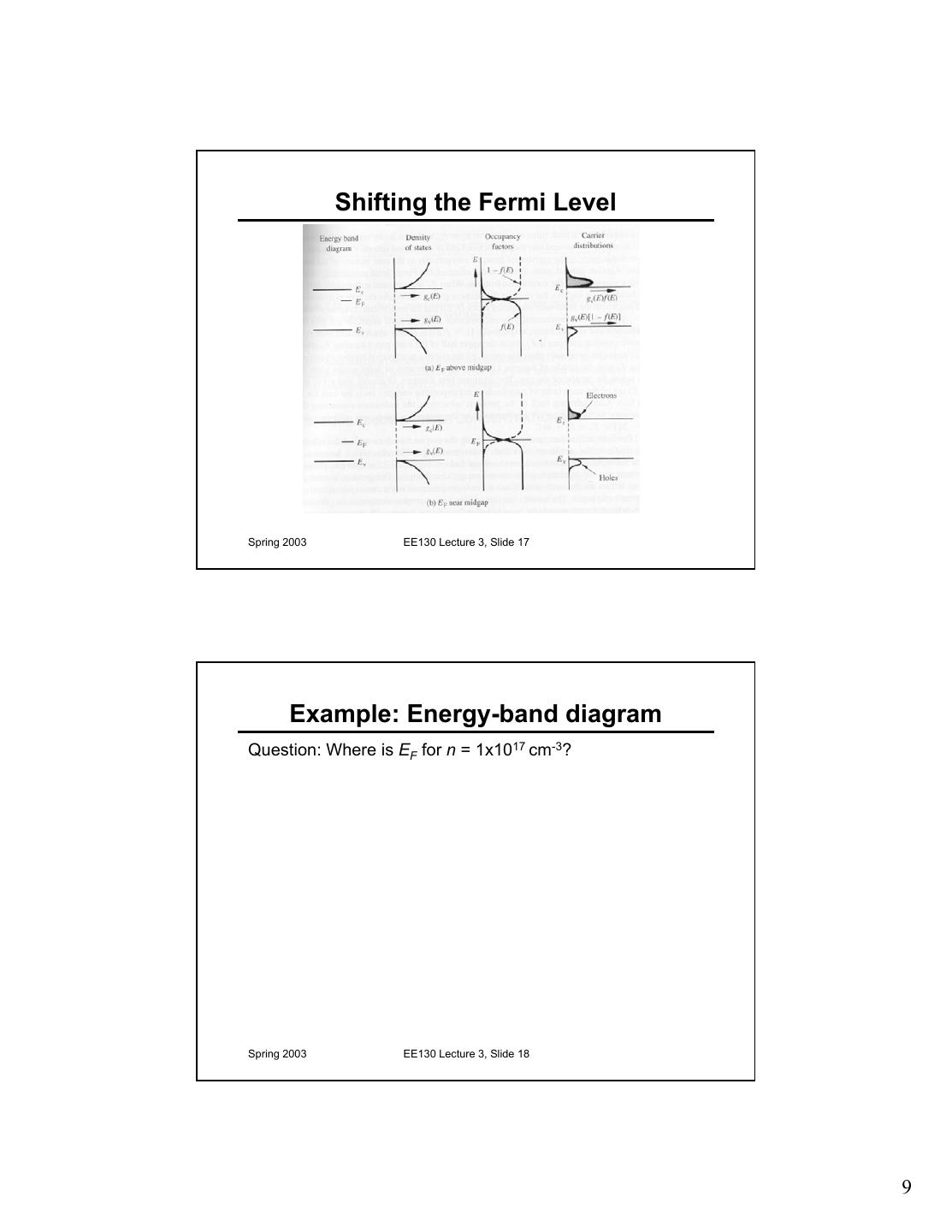
9 . Shifting the Fermi Level
Spring 2003 EE130 Lecture 3, Slide 17
Example: Energy-band diagram
Question: Where is EF for n = 1x1017 cm-3?
Spring 2003 EE130 Lecture 3, Slide 18
9
10 .Carrier Concentration vs. Temperature
intrinsic regime
n = ND
ln n
“freeze-out” regime
1/T
high room cryogenic
temp. temperature temperature
Spring 2003 EE130 Lecture 3, Slide 19
Dependence of EF on Temperature
Ec
300K
400K
ed
nor-dop
Ef, Do
Ef , Acce
ptor-dop
ed
400K
300K
Ev
1013 1014 1015 1016 1017 1018 1019 1020
N A or N D (cm-3)
Spring 2003 EE130 Lecture 3, Slide 20
10
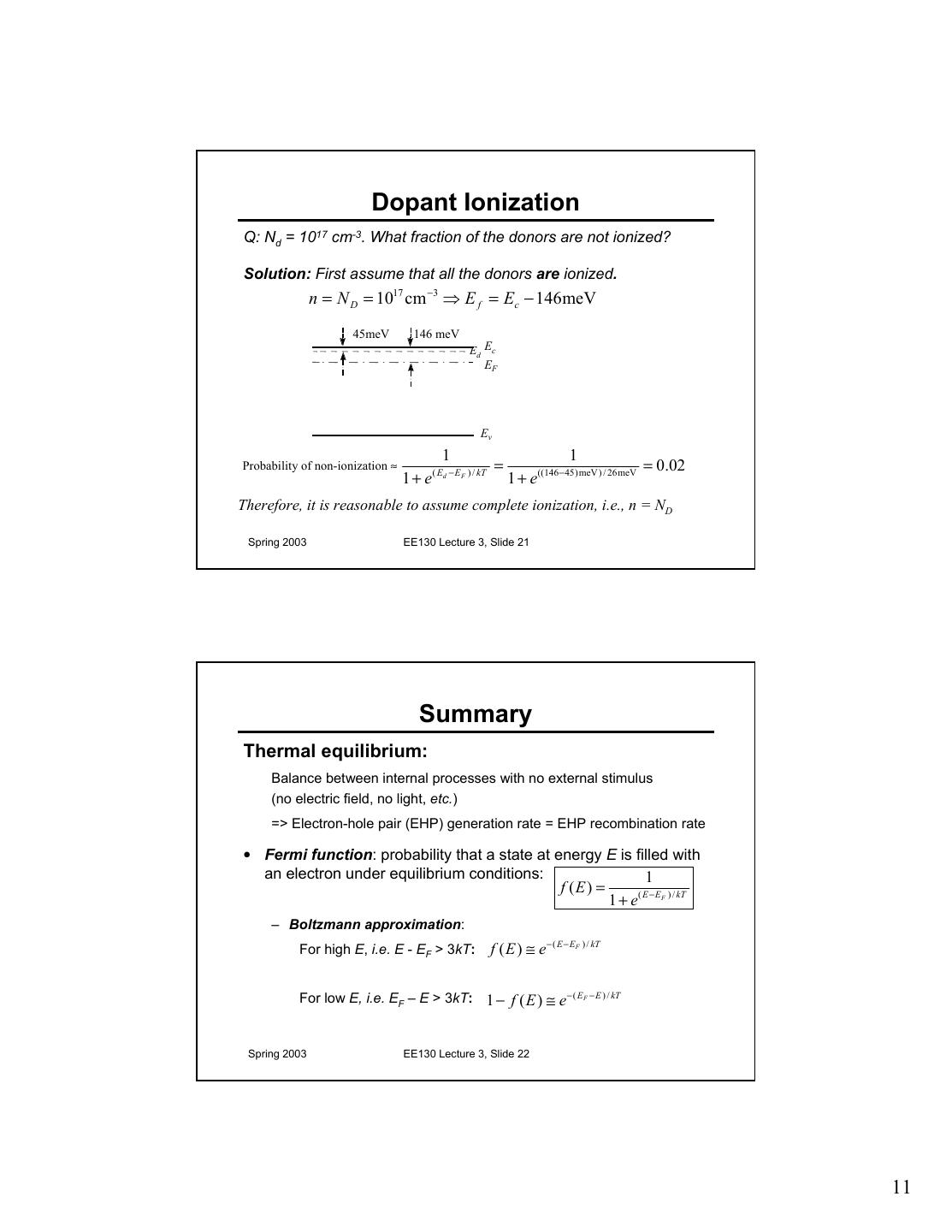
11 . Dopant Ionization
Q: Nd = 1017 cm-3. What fraction of the donors are not ionized?
Solution: First assume that all the donors are ionized.
n = N D = 1017 cm −3 ⇒ E f = Ec − 146meV
45meV 146 meV
Ed Ec
EF
Ev
1 1
Probability of non-ionization ≈ = = 0.02
1 + e( Ed − EF ) / kT 1 + e((146−45) meV ) / 26 meV
Therefore, it is reasonable to assume complete ionization, i.e., n = ND
Spring 2003 EE130 Lecture 3, Slide 21
Summary
Thermal equilibrium:
Balance between internal processes with no external stimulus
(no electric field, no light, etc.)
=> Electron-hole pair (EHP) generation rate = EHP recombination rate
• Fermi function: probability that a state at energy E is filled with
an electron under equilibrium conditions: 1
f (E) = ( E − E F ) / kT
1+ e
– Boltzmann approximation:
For high E, i.e. E - EF > 3kT: f ( E ) ≅ e − ( E − EF ) / kT
For low E, i.e. EF – E > 3kT: 1 − f ( E ) ≅ e − ( E F − E ) / kT
Spring 2003 EE130 Lecture 3, Slide 22
11
12 . n = N c e − ( Ec − EF ) / kT = ni e( EF − Ei ) / kT
p = N v e − ( EF − Ev ) / kT = ni e( Ei − EF ) / kT
Spring 2003 EE130 Lecture 3, Slide 23
12
热门城市
