展开查看详情
1 . Lecture #25
• Design Project:
– Due in class (5 PM) on Thursday May 1st
• 20 pt penalty for late submissions, accepted until 5 PM
on 5/8
– Your BJT design does not need to meet the
performance specifications when WB and NB are varied
by +/- 10%
– Equation for ∆EG assumes NE is in cm-3 and T is in K
EE130 QUIZ SCORE TREND
• Quiz#5 Results:
30
(undergrad.’s only)
21.6 20.5
20 18.9
N=60 17.9 17.9
10
Mean=20.5
0
Std.Dev.=3.6
Q1 Q2 Q3 Q4 Q5
Spring 2003 EE130 Lecture 25, Slide 1
OUTLINE
• NMOSFET I-V
• Effective mobility
• Transconductance
• PMOSFET I-V
• Subthreshold current
Spring 2003 EE130 Lecture 25, Slide 2
1
2 . Ideal MOSFET I-V Characteristics
(Enhancement Mode NMOS Transistor)
Saturation
Linear region
region
Spring 2003 EE130 Lecture 25, Slide 3
Review: Qualitative Operation of the NMOSFET
depletion layer
The potential barrier to electron flow from the source
into the channel is lowered by applying VGS> VT
Electrons flow from the
source to the drain by drift,
when VDS>0. (IDS > 0.)
The channel potential
varies from VS at the
source end to VD at the
drain end.
(The inversion layer can be
Spring 2003 EE130 Lecture 25, Slide 4 modeled as a resistor.)
2
3 . When VD is increased to be equal to
VG-VT, the inversion-layer charge
density at the drain end of the
channel equals zero, i.e. the
channel becomes “pinched off”
As VD is increased above VG-VT, the
length ∆L of the “pinch-off” region
increases. The voltage applied
across the inversion layer is always
VDsat=VGS-VT, and so the current
saturates:
I Dsat = I DS V
DS =VDsat
If ∆L is significant compared to L, then
IDS will increase slightly with increasing
VDS>VDsat, due to “channel-length
modulation”
Spring 2003 EE130 Lecture 25, Slide 5
NMOSFET I-V Characteristics
• VD > VS
• Current in the channel flows by drift
• Channel voltage VC(y) varies continuously between
the source and the drain
2qN Aε Si ( 2ψ B + VCB ( y ))
VT = VFB + VC ( y ) + 2ψ B +
Cox
• Channel inversion charge
Q ( y)
Qinv ( y ) = −Coxe VG − VFB − VC ( y ) − 2ψ B − dep
Coxe
W
Spring 2003 EE130 Lecture 25, Slide 6
3
4 . 1st-Order Approximation
• Neglect variation of Qdep with y
Qdep = 2qN Aε Si (2ψ B + VSB )
⇒ Qinv = −Coxe [VG − VT + VS − VC ]
where VT = threshold voltage at the source end:
2qN Aε Si ( 2ψ B + VSB )
VT = VFB + VS + 2ψ B +
Cox
Spring 2003 EE130 Lecture 25, Slide 7
NMOSFET Current (1st-order approx.)
• Consider an incremental length dy in the channel.
The voltage drop across this region is
dy dy I dy
dVC = I DS dR = I DS = I DS = − DS
σWTinv qµeff nWTinv Qinv µeff W
L VD
∫I
0
DS dy = − ∫ µeff WQinv (VC )dVC
VS
W VD
I DS = − µeff ∫ Qinv (VC )dVC
L VS
W V
I DS = µeff Coxe VGS − VT − DS VDS in the linear region
L 2
W
I DS = I Dsat = Coxe µeff (VGS − VT ) 2 in the saturation region
2L
Spring 2003 EE130 Lecture 25, Slide 8
4
5 . Effective Mobility
V
I DS = WQinv v = WQ inv µeff E = WQ inv µeff DS
L
= (W / L) µeff Coxe (VG − VT )VDS
where µeff is the effective electron mobility
The NMOSFET can be modelled as a resistor at low VDS:
VDS L
RDS = =
I DS Wµeff Coxe (VG − VT )
Spring 2003 EE130 Lecture 25, Slide 9
µeff vs. Effective Normal Field
(Vgs + V t + 0.2)/6Toxe (MV/cm)
(NFET) Scattering mechanisms:
• coulombic scattering
• phonon scattering
• surface roughness
scattering
(PFET)
–(Vgs + 1.5V t – 0.25)/6Tox e (MV/cm)
Spring 2003 EE130 Lecture 25, Slide 10
5
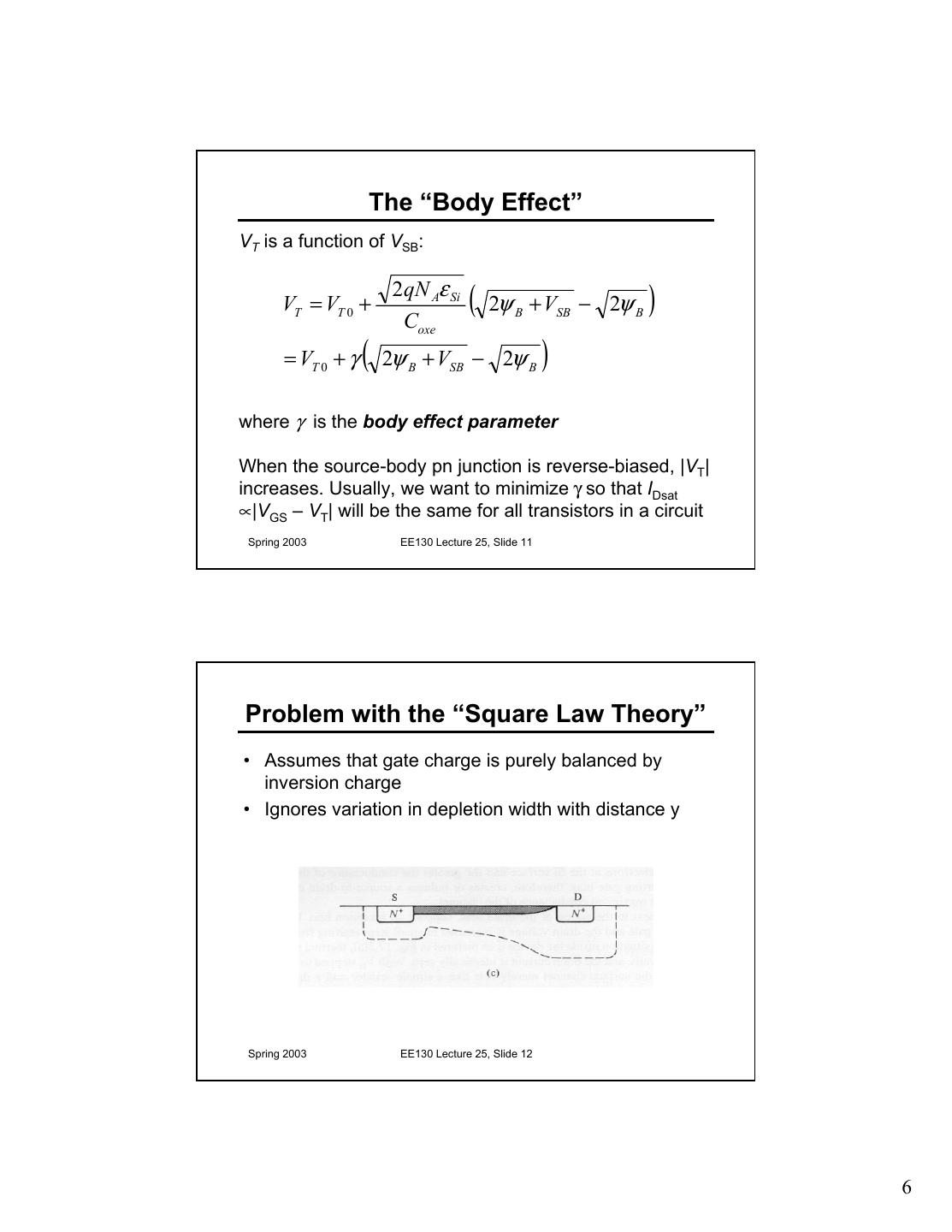
6 . The “Body Effect”
VT is a function of VSB:
VT = VT 0 +
2qN Aε Si
Coxe
( 2ψ B + VSB − 2ψ B )
= VT 0 + γ ( 2ψ B + VSB − 2ψ B )
where γ is the body effect parameter
When the source-body pn junction is reverse-biased, |VT|
increases. Usually, we want to minimize γ so that IDsat
∝|VGS – VT| will be the same for all transistors in a circuit
Spring 2003 EE130 Lecture 25, Slide 11
Problem with the “Square Law Theory”
• Assumes that gate charge is purely balanced by
inversion charge
• Ignores variation in depletion width with distance y
Spring 2003 EE130 Lecture 25, Slide 12
6
7 . Modified Model
W m
I DS = Coxe µeff (VGS − VT − VDS )VDS
L 2
Cdm 3T
where m = 1 + = 1 + oxe since ε Si = 3ε Si O2
Coxe Wdm
Spring 2003 EE130 Lecture 25, Slide 13
Modified Model: IDsat & Transconductance
• saturation region:
V − VT
VD ≥ VDsat = GS
m
W
I Dsat = Coxe µeff (VGS − VT )2
2mL
• transconductance: gm= dIDS/dVGS
W
g msat = Coxe µ eff (VGS − VT )
mL
Spring 2003 EE130 Lecture 25, Slide 14
7
8 . MOSFET VT Measurement
• VT can be determined by plotting IDS vs. VGS,
using a low value of VDS
Spring 2003 EE130 Lecture 25, Slide 15
P-Channel MOSFET
• The PMOSFET turns on when VGS < VTp
– Holes flow from SOURCE to DRAIN
⇒ DRAIN is biased at a lower potential than the SOURCE
VG
• VDS < 0
VS VD
GATE IDS • IDS < 0
P+ P+ • |IDS| increases with
N • |VGS - VTp|
• |VDS| (linear region)
VB
• In CMOS technology, the threshold voltages
are usually symmetric: VTp = -VTn
Spring 2003 EE130 Lecture 25, Slide 16
8
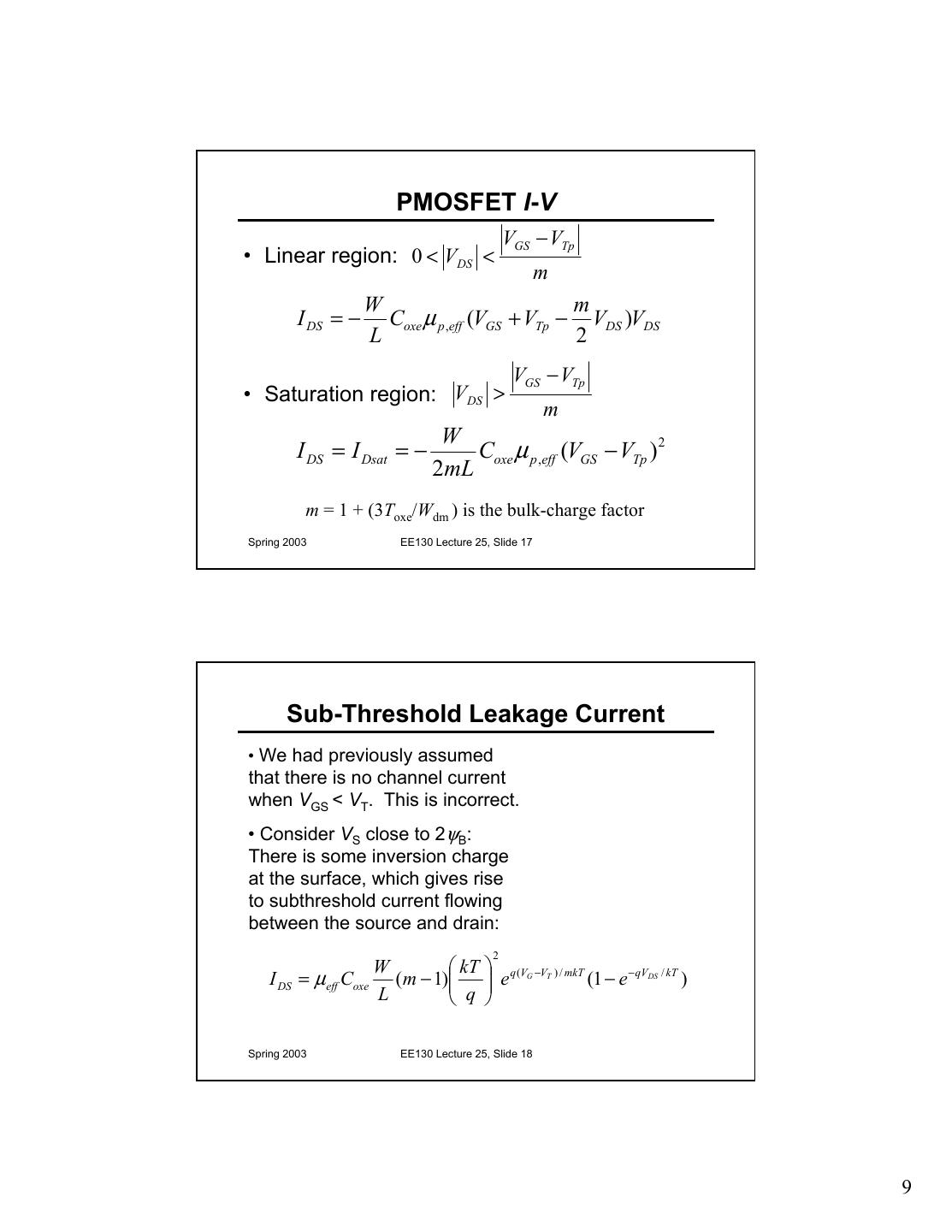
9 . PMOSFET I-V
VGS − VTp
• Linear region: 0 < VDS <
m
W m
I DS = − Coxe µ p ,eff (VGS + VTp − VDS )VDS
L 2
VGS − VTp
• Saturation region: VDS >
m
W
I DS = I Dsat = − Coxe µ p ,eff (VGS − VTp ) 2
2mL
m = 1 + (3Toxe/Wdm ) is the bulk-charge factor
Spring 2003 EE130 Lecture 25, Slide 17
Sub-Threshold Leakage Current
• We had previously assumed
that there is no channel current
when VGS < VT. This is incorrect.
• Consider VS close to 2ψB:
There is some inversion charge
at the surface, which gives rise
to subthreshold current flowing
between the source and drain:
2
W kT q (VG −VT ) / mkT
I DS = µeff Coxe ( m − 1) e (1 − e − qVDS / kT )
L q
Spring 2003 EE130 Lecture 25, Slide 18
9
10 . Sub-Threshold Slope S
−1
d (log10 I DS )
S ≡
dV GS
kT C
= ln(10)(1 + dm )
q Coxe
Spring 2003 EE130 Lecture 25, Slide 19
VT Design Tradeoff
Spring 2003 EE130 Lecture 25, Slide 20
10
热门城市
