展开查看详情
1 . Lecture #10
ANNOUNCEMENT
– Quiz #2 next Thursday (2/27) covering
• carrier action (drift, diffusion, R-G)
• continuity & minority-carrier diffusion equations
• metal-semiconductor contacts
OUTLINE
• pn junction electrostatics
Reading: Chapter 5
Spring 2003 EE130 Lecture 10, Slide 1
pn Junctions
Donor s
N- type
P- type
I – V +
I
N P
V
Reverse bias Forward bias diode
symbol
Spring 2003 EE130 Lecture 10, Slide 2
1
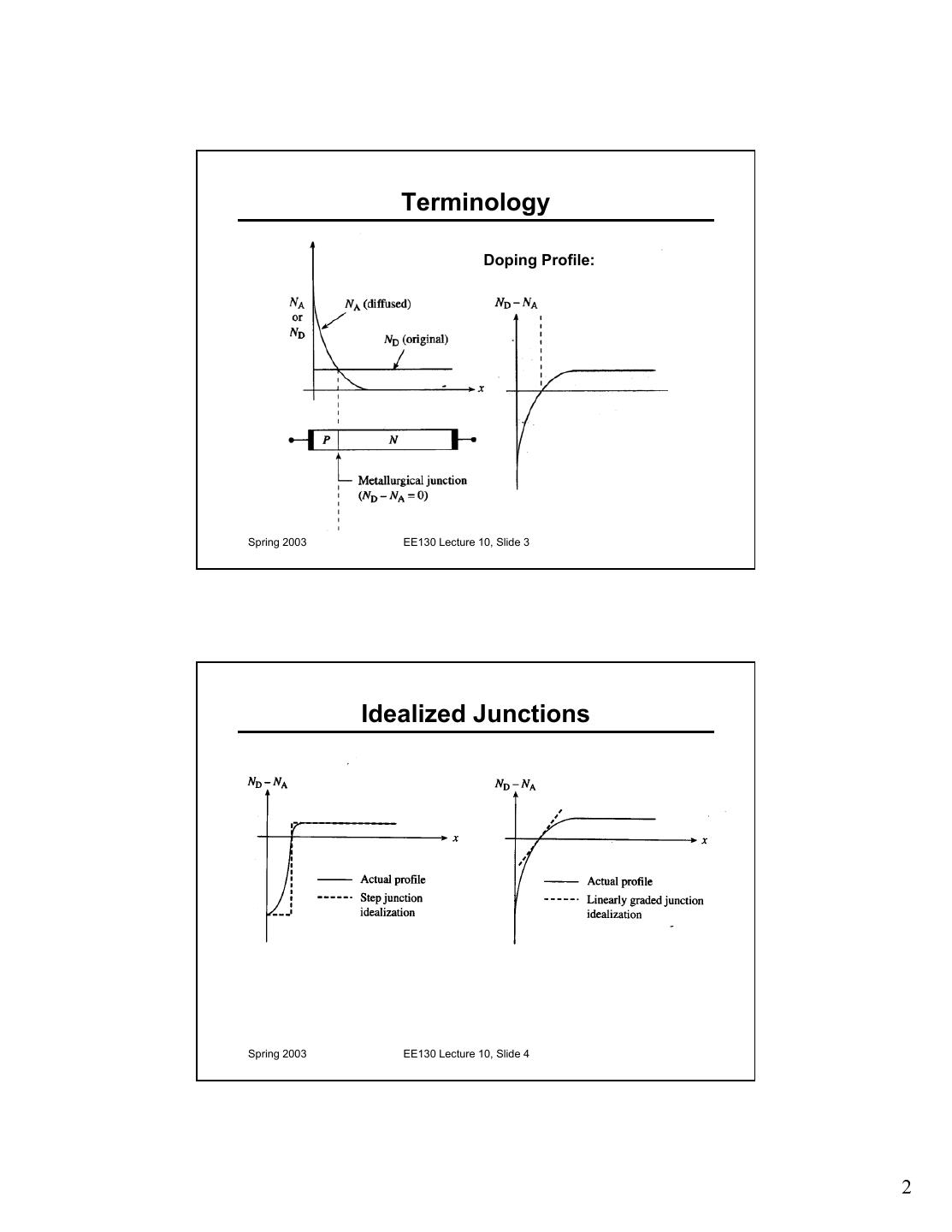
2 . Terminology
Doping Profile:
Spring 2003 EE130 Lecture 10, Slide 3
Idealized Junctions
Spring 2003 EE130 Lecture 10, Slide 4
2
3 . Energy Band Diagram
Spring 2003 EE130 Lecture 10, Slide 5
Qualitative Electrostatics
Band diagram
Electrostatic potential
Electric field
Charge density
Spring 2003 EE130 Lecture 10, Slide 6
3
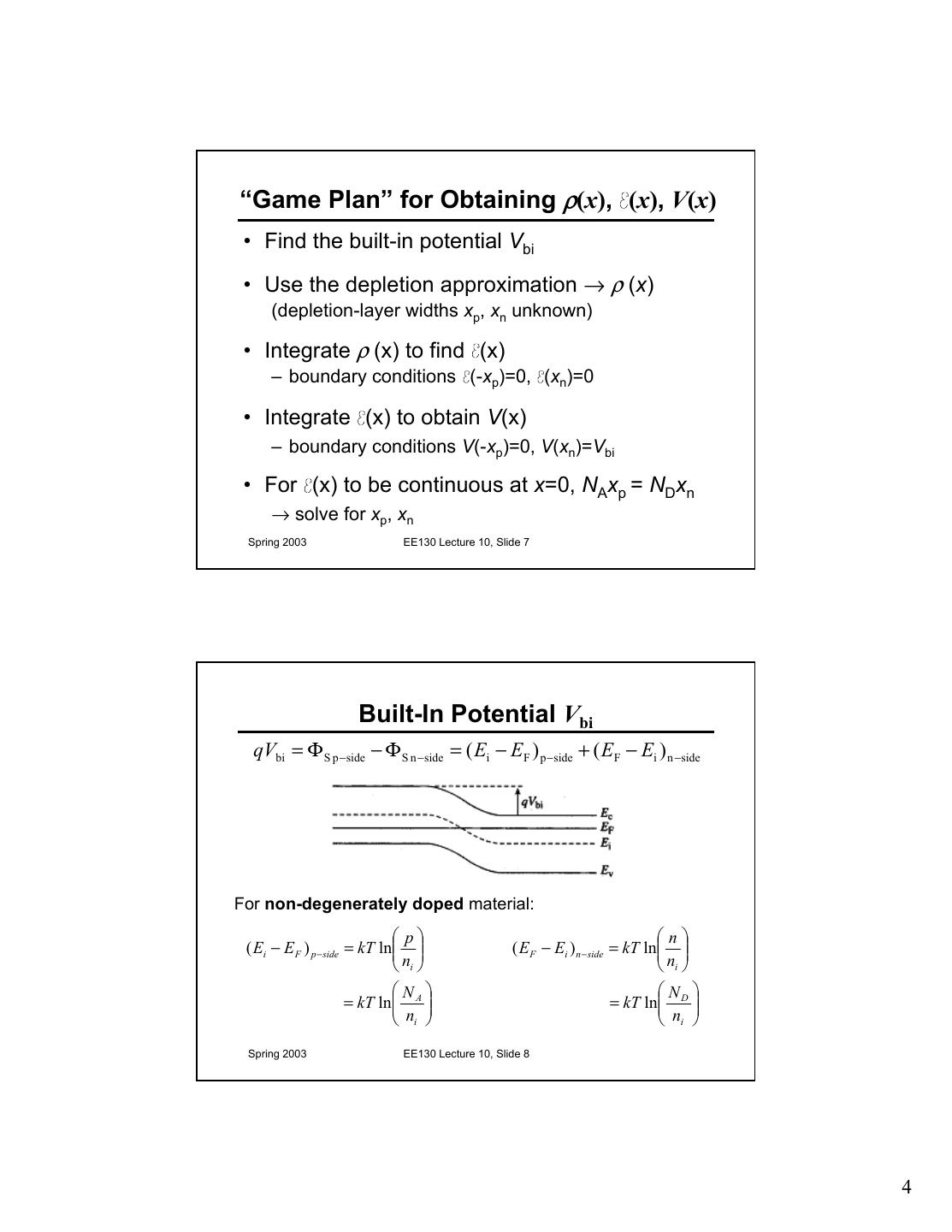
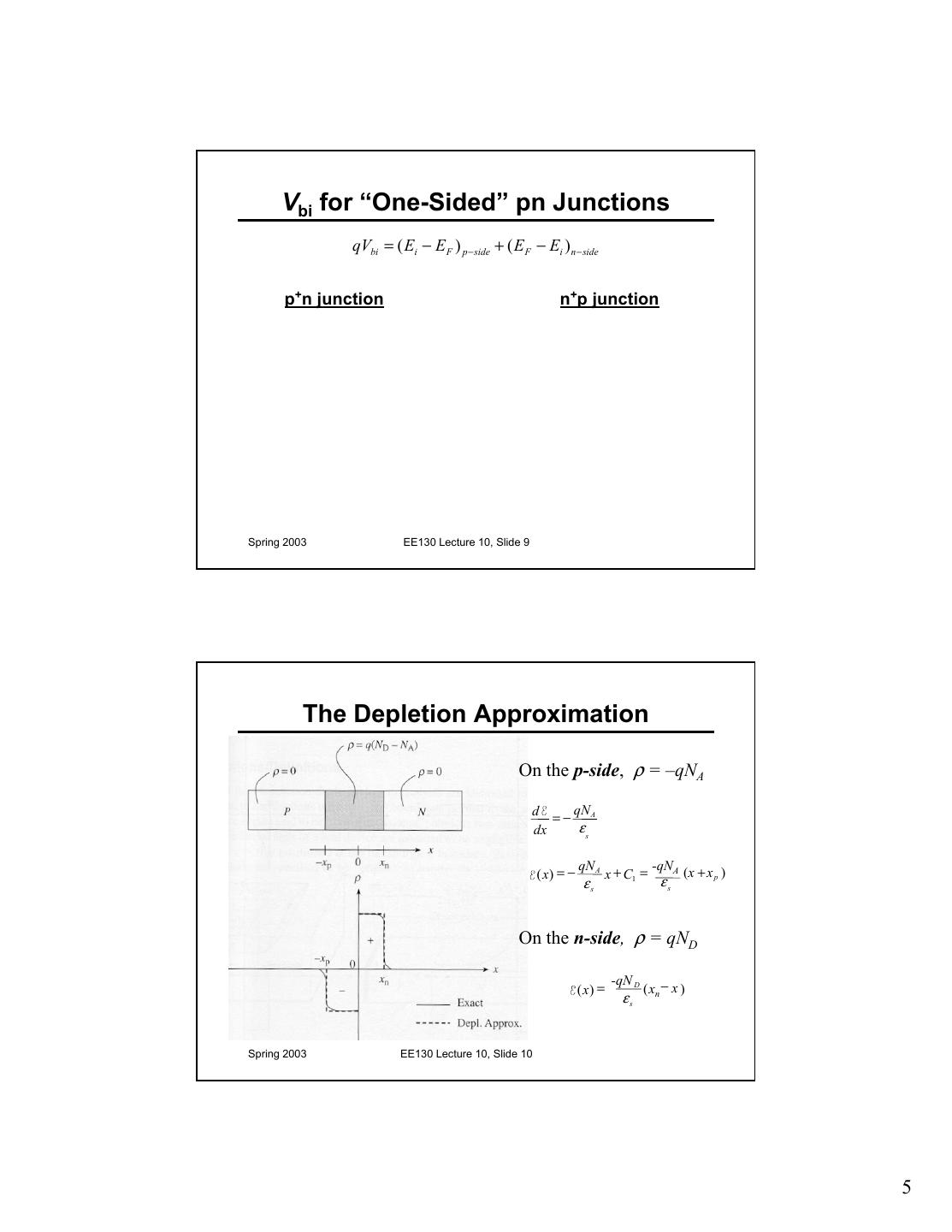
4 .“Game Plan” for Obtaining ρ(x), (x), V(x)
• Find the built-in potential Vbi
• Use the depletion approximation → ρ (x)
(depletion-layer widths xp, xn unknown)
• Integrate ρ (x) to find (x)
– boundary conditions (-xp)=0, (xn)=0
• Integrate (x) to obtain V(x)
– boundary conditions V(-xp)=0, V(xn)=Vbi
• For (x) to be continuous at x=0, NAxp = NDxn
→ solve for xp, xn
Spring 2003 EE130 Lecture 10, Slide 7
Built-In Potential Vbi
qVbi = Φ S p −side − Φ S n −side = ( Ei − EF ) p −side + ( EF − Ei ) n −side
For non-degenerately doped material:
p n
( Ei − E F ) p − side = kT ln ( E F − Ei ) n − side = kT ln
ni ni
N N
= kT ln A = kT ln D
ni ni
Spring 2003 EE130 Lecture 10, Slide 8
4
5 . Vbi for “One-Sided” pn Junctions
qVbi = ( Ei − E F ) p − side + ( E F − Ei ) n − side
p+n junction n+p junction
Spring 2003 EE130 Lecture 10, Slide 9
The Depletion Approximation
On the p-side, ρ = –qNA
d qN
=− A
dx εs
qN A -qNA
( x) = − x + C1 = (x + x p )
εs εs
On the n-side, ρ = qND
-qN D
( x) = ( xn − x )
εs
Spring 2003 EE130 Lecture 10, Slide 10
5
6 . Electric Field in the Depletion Layer
The electric field is continuous at x = 0
⇒ NAxp = NDxn
Spring 2003 EE130 Lecture 10, Slide 11
Electrostatic Potential in the Depletion Layer
On the p-side:
qN A
V ( x) = ( x + x p ) 2 + D1
2ε s
(arbitrarily choose the voltage at x = xp to be 0)
On the n-side:
qN D qN D
V ( x) = − ( xn − x ) 2 + D2 = Vbi − ( xn − x ) 2
2ε s 2ε s
Spring 2003 EE130 Lecture 10, Slide 12
6
7 .• At x = 0, expressions for p-side and n-side must be equal:
• We also know that NAxp = NDxn
Spring 2003 EE130 Lecture 10, Slide 13
Depletion Layer Width
• Eliminating xp, we have:
2ε sVbi NA
xn =
q ND (N A + ND )
• Eliminating xn, we have:
2ε sVbi ND
xp =
q N A(N A + ND )
• Summing, we have:
2ε sVbi 1 1
xn + x p = W = +
q N A ND
Spring 2003 EE130 Lecture 10, Slide 14
7
8 . One-Sided Junctions
If NA >> ND as in a p+n junction:
2ε sVbi
W= ≈ xn
qN D
x p = xn N D N A ≅ 0
What about a n+p junction?
1 1 1 1
W = 2ε s Vbi qN where = + ≈
N N D N A lighter dopant density
Spring 2003 EE130 Lecture 10, Slide 15
Peak Electric Field
∫ ε dx = 2 ε (0) W = Vbi − VA
1
2ε s
• For a one-sided junction, W ≅ (Vbi − VA )
qN
so ε (0) = 2(V W− V ) ≅
bi A 2qN (Vbi − VA )
εs
Spring 2003 EE130 Lecture 10, Slide 16
8
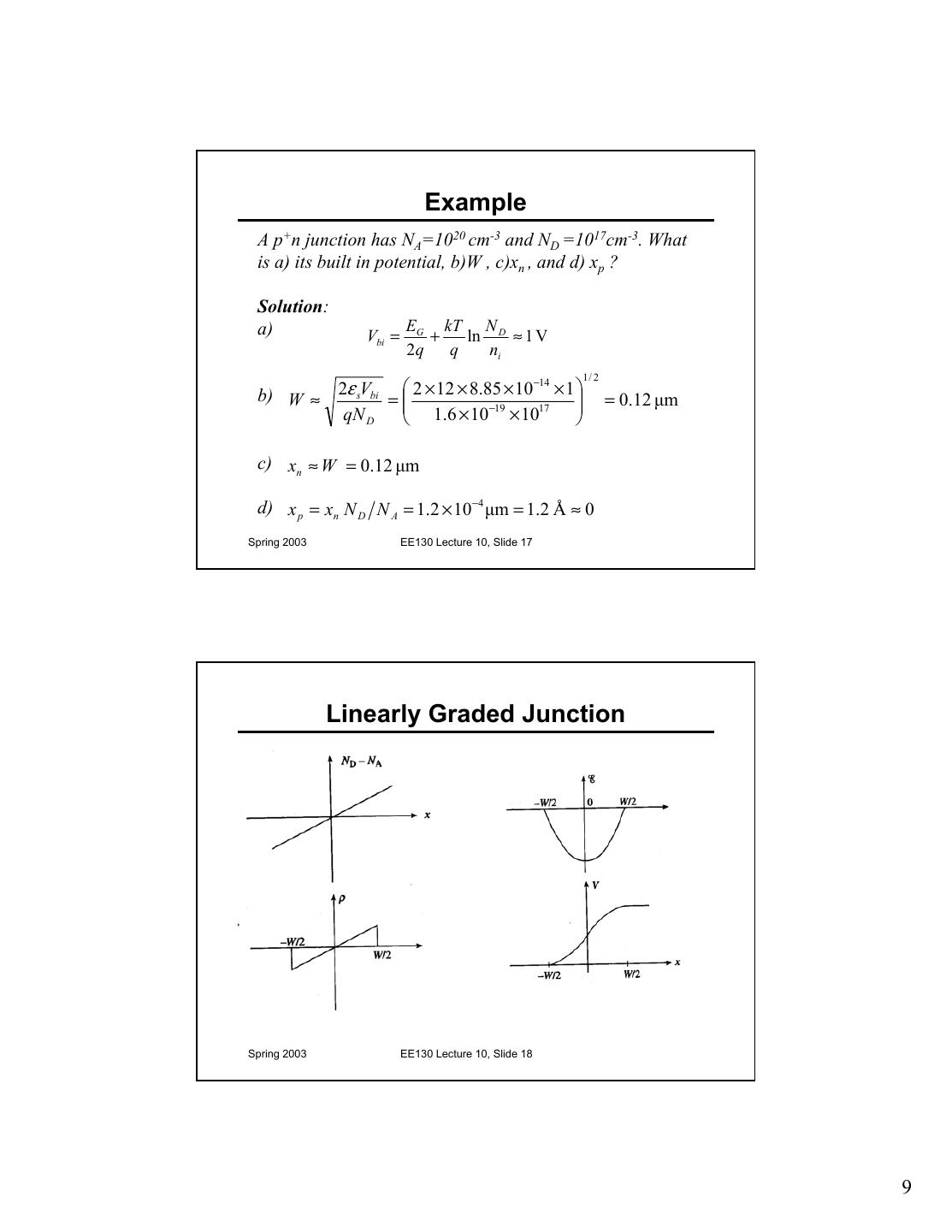
9 . Example
A p+n junction has NA=1020 cm-3 and ND =1017cm-3. What
is a) its built in potential, b)W , c)xn , and d) xp ?
Solution:
a) EG kT N D
Vbi = + ln ≈1V
2q q ni
1/ 2
b) W ≈ 2ε sVbi = 2 × 12 × 8.85 × 10 × 1
−14
1.6 × 10−19 × 1017 = 0.12 µm
qN D
c) xn ≈ W = 0.12 µm
d) x p = xn N D N A = 1.2 × 10−4 µm = 1.2 Å ≈ 0
Spring 2003 EE130 Lecture 10, Slide 17
Linearly Graded Junction
Spring 2003 EE130 Lecture 10, Slide 18
9
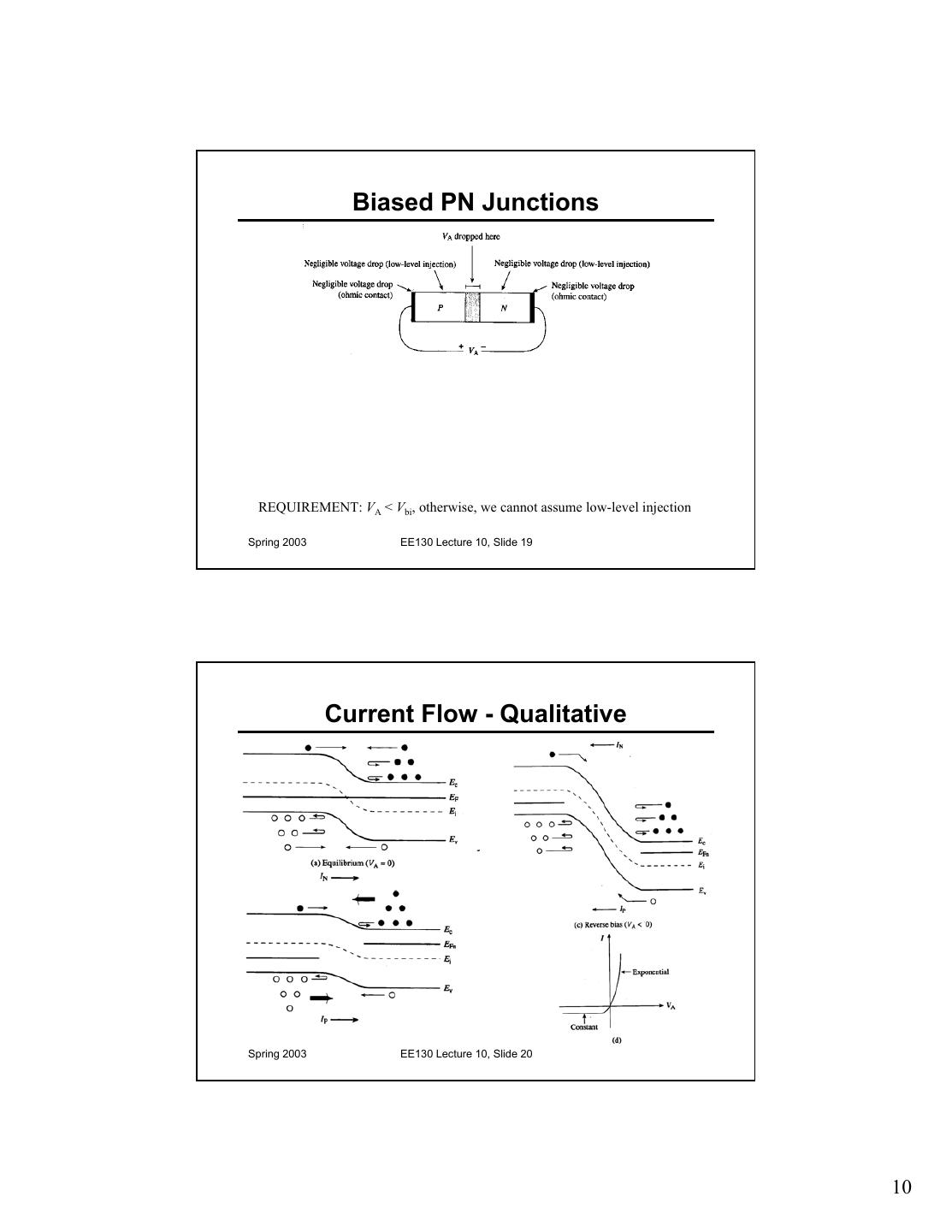
10 . Biased PN Junctions
REQUIREMENT: VA < Vbi, otherwise, we cannot assume low-level injection
Spring 2003 EE130 Lecture 10, Slide 19
Current Flow - Qualitative
Spring 2003 EE130 Lecture 10, Slide 20
10
11 . Effect of Bias on Electrostatics
Spring 2003 EE130 Lecture 10, Slide 21
11
热门城市
