连续介质方程,少数载流子扩散方和费米能级

1 . Lecture #7 Quiz #1 Results (undergrad. scores only) N = 73; mean = 21.6; σ = 2.1; high = 25; low = 14 OUTLINE – Continuity equations – Minority carrier diffusion equations – Quasi-Fermi levels Reading: Chapter 3.4, 3.5 Spring 2003 EE130 Lecture 7, Slide 1 Clarification: Direct vs. Indirect Band Gap Small change in momentum Large change in momentum required for recombination required for recombination Æ momentum is conserved by Æ momentum is conserved by photon emission phonon + photon emission Spring 2003 EE130 Lecture 7, Slide 2 1 

2 .Example: Relaxation to Equilibrium State Consider a semiconductor with no current flow in which thermal equilibrium is disturbed by the sudden creation of excess holes and electrons. The system will relax back to the equilibrium state via R-G mechanism: dn ∆n =− for electrons in p-type material dt τn dp ∆p =− for holes in n-type material dt τp Spring 2003 EE130 Lecture 7, Slide 3 Net Recombination Rate (General Case) • For arbitrary injection levels and both carrier types in a non-degenerate semiconductor, the net rate of recombination is: d∆n d∆p pn − ni2 = =− dt dt τ p (n + n1 ) + τ n ( p + p1 ) where n1 ≡ ni e( ET − Ei ) / kT and p1 ≡ ni e( Ei − ET ) / kT Spring 2003 EE130 Lecture 7, Slide 4 2 

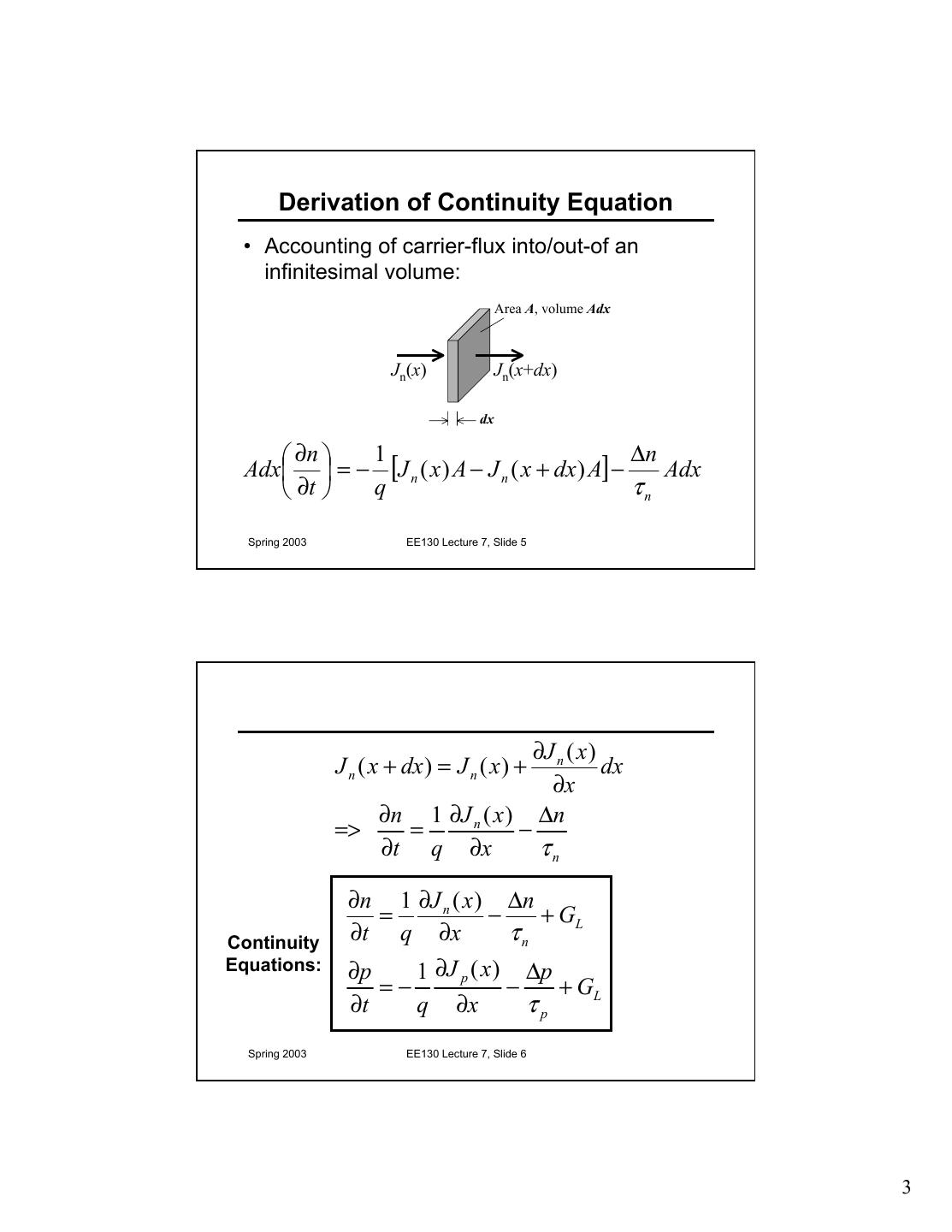
3 . Derivation of Continuity Equation • Accounting of carrier-flux into/out-of an infinitesimal volume: Area A, volume Adx Jn(x) Jn(x+dx) dx  ∂n  1 ∆n Adx  = − [J n ( x ) A − J n ( x + dx ) A] − Adx ∂  t q τ n Spring 2003 EE130 Lecture 7, Slide 5 ∂J n ( x ) J n ( x + dx ) = J n ( x ) + dx ∂x ∂n 1 ∂J n ( x ) ∆n => = − ∂t q ∂x τn ∂n 1 ∂J n ( x ) ∆n = − + GL Continuity ∂t q ∂x τn Equations: ∂p 1 ∂J p ( x ) ∆p =− − + GL ∂t q ∂x τp Spring 2003 EE130 Lecture 7, Slide 6 3 

4 .Derivation of Minority-Carrier Diffusion Equations • Simplifying assumptions: – 1-D – negligible electric field – n0, p0 are independent of x – low-level injection conditions Spring 2003 EE130 Lecture 7, Slide 7 Minority Carrier Diffusion Length • Consider the special case: – Constant minority-carrier (hole) injection at x=0 – Steady state, no light d 2 ∆p ∆p ∆p = = 2 dx 2 DPτ p LP LP is the hole diffusion length L p ≡ D pτ p Spring 2003 EE130 Lecture 7, Slide 8 4 

5 .• Physically, LP and LN represent the average distance that minority carriers can diffuse into a sea of majority carriers before being annihilated. • Example: ND=1016 cm-3; τp = 10-6 s Spring 2003 EE130 Lecture 7, Slide 9 Quasi-Fermi Levels • Whenever ∆n = ∆p ≠ 0, np ≠ ni2. However, we would like to preserve and use the relations: n = N c e − ( Ec − EF ) / kT p = N v e − ( EF − Ev ) / kT • These equations imply np = ni2, however. The solution is to introduce two quasi-Fermi levels FN and FP such that n = N c e − ( Ec − FN ) / kT p = N v e − ( FP − Ev ) / kT Spring 2003 EE130 Lecture 7, Slide 10 5 

6 . Example: Quasi-Fermi Levels Consider a Si sample with ND = 1017 cm-3 and ∆n = ∆p = 1014 cm-3. (a) Find n: n = n0+ ∆n = ND + ∆n ≈ 1017 cm-3 (b) Find p: p = p0+ ∆p = ( ni2 / ND ) + ∆p ≈ 1014 cm-3 (c) Find the np product: np ≈ 1017 × 1014 = 1031 cm-6 >> ni2 Spring 2003 EE130 Lecture 7, Slide 11 (d) Find FN: − ( E − F ) / kT n = 1017 cm-3 = N c e c N Ec- FN = kT × ln(Nc/1017) = 0.026 eV × ln(2.8×1019/1017) = 0.15 eV (e) Find FP: − ( F − E ) / kT p = 1014 cm-3 = N v e P v FP–Ev = kT × ln(Nv/1017) = 0.026 eV × ln(1019/1014) = 0.30 eV Spring 2003 EE130 Lecture 7, Slide 12 6